Question

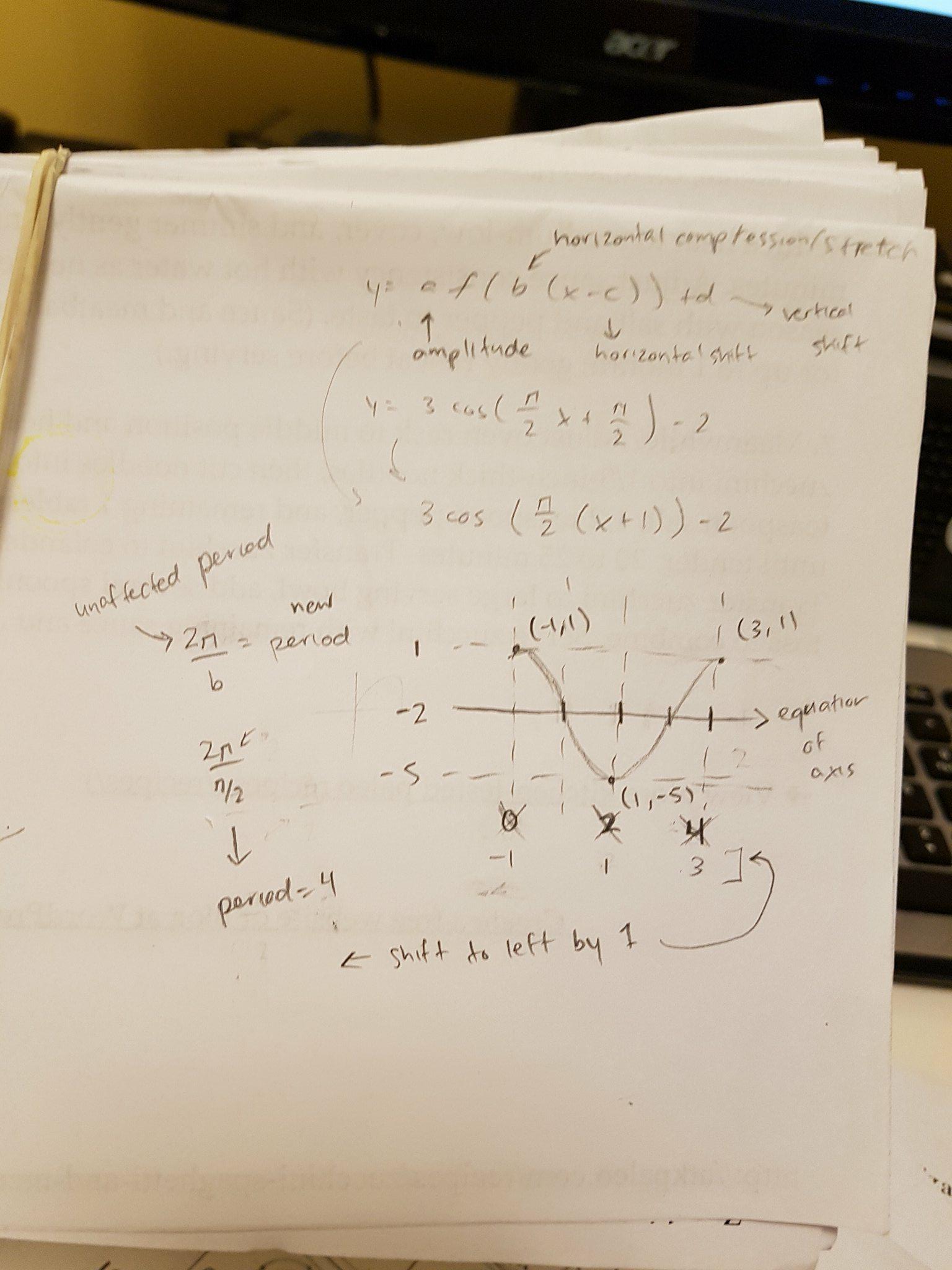
How do you find the period, amplitude and sketch `y=3cos((pix)/2+pi/2)-2`?

Answer 1

Sorry I'm not a committed teacher, (just using this site for my own question). You want to first understand the properties of the standard equation for a sinusoidal function (for cosine, and sine).

Explanation:

I'm not sure how much you know, but I'll try to briefly explain things. It's midnight for me, lol:

`y = a f (b (x-c))+d`

`a` is the amplitude
`f` is the function (cos in this case)
`b` is the horizontal compression or stretch
`c` is horizontal shift
`d` is the vertical shift

So right off the bat, you have your amplitude: 3.

Next I would suggest creating a rough sketch using the `d` value, which is going to be your equation of axis (the centre between the peaks and valleys).

Using your amplitude, this is how much you will add (for the peak) and subtract (for the valley) to the equation of axis.

Then you need to factor out a `b` value from the x, so the x is isolated with a `c` value.

To find the period, since we're in radians, you would take `(2pi)/b` and that is your period.

Then based on the `c` value (horizontal shift) you can adjust your values accordingly.

Once again this is a rough answer, hopefully someone better will come along to help you. Here is rough work though: