Question

A triangle has sides A, B, and C. Sides A and B are of lengths `4` and `5`, respectively, and the angle between A and B is `pi/4`. What is the length of side C?

Answer 1

`≈` `"3.566 units"`

Explanation:

In this case, we can use the law of cosines. The law of cosines works when you have any triangle, and you know the value of two side lengths and one angle in between (which would be the case in this problem). Using the formula:

`a^2 = b^2 + c^2 - 2*b*c*cos(theta)`

Where `a` is the length that we want to find, `b` and `c` are the side lengths that we already know, and `theta` is the angle that we already know. We end up with

`a^2 = 4^2 + 5^2 - 2(4)(5)cos(π/4)`

`a^2 = 16 + 25 - 40(sqrt(2)/2)`

`a^2 = 12.715...`

`a ≈ 3.566`

Answer 2

`c=3.5665`

Explanation:

According to the law of cosines, `c^2=a^2+b^2-2abcosC` where the small letters are side lengths and big letters are the angles opposite the respective side length.

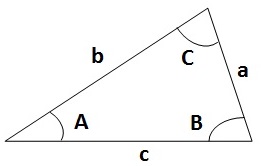
We know that `/_C` is `pi/4`, `a=4`, and `b=5`. With that information, we can solve for `c`.

`c^2=4^2+5^2-2*4*5*cos (pi/4)`
`c^2=16+25-40*0.707`
`c^2=41-28.28`
`c^2=12.72`
`c=3.5665`