Question

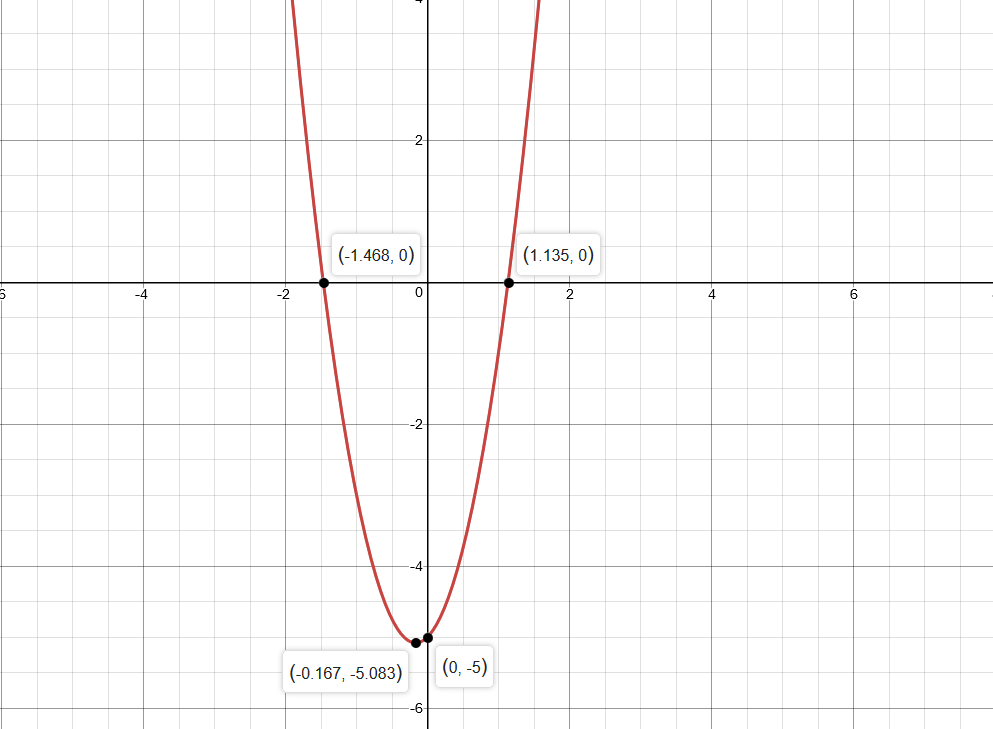
How do you identify the important parts of `f(x)= 3x²+x-5` to graph it?

Answer 1

Vertex`(-1/6, -61/12)`
y-intercept`(0,-5)`
X-intercepts `((sqrt61-1)/6, 0); (-(sqrt61-1)/6, 0)`

Explanation:

Given -

`f(x)=3x^2+x-5`

`y=3x^2+x-5`

Vertex

`x=(-b)/(2a)=(-1)/(2xx3)=-1/6`

At `x=-1/6;y= 3(-1/6)^2+(-1/6)-5`

`y=1/12-1/6-5=(1-2-60)/12=61/12=-61/4`

Vertex`(-1/6, -61/12)`

y-intercept
At`x=0; y=3(0)^2+(0)-5=5`

y-intercept#(0,-5)

x-intercepts

`3x^2+x=5`

`x^2+1/3x=5/3`

`x^2+1/3x+1/36=5/3+1/36=(60+1)/36=61/36`

`(x+1/6)^2=61/36`

`x+1/6=+-sqrt(61/36)=+-sqrt61/6`

`x=sqrt(61/6)-1/6=(sqrt61-1)/6`

`x=-sqrt(61/36)-1/6=-(sqrt61-1)/6`

X-intercepts `((sqrt61-1)/6, 0); (-(sqrt61-1)/6, 0)`