Question

Question #07e8f

Answer 1

The reaction is second-order.

Explanation:

You're dealing with a decomposition reaction of the form

`"AB " -> " products"`

for which the differential rate law can be written as

`"rate" = k * ["AB"]^n`

Here

`k` - the rate constant for the reaction
`n` - the order of the reaction

According to experimental data, you have

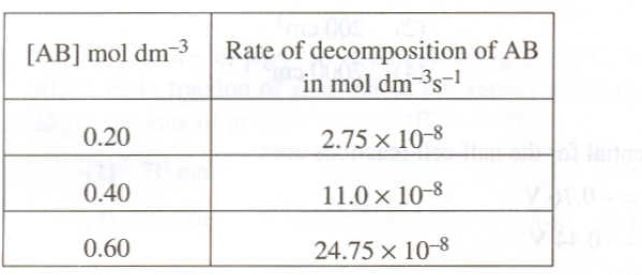
Pick any two entries and plug the data into the differential rate law

`2.75 * 10^(-8)"mol dm"^(-3)"s"^(-1) = k * ("0.20 mol dm"^(-3))^n`

`11.0 * 10^(-8) "mol dm"^(-3)"s"^(-1) = k * ("0.40 mol dm"^(-3)")^n`

You can get rid of the unknown rate constant by dividing these two equations

`(2.73 * color(red)(cancel(color(black)(10^(-8)))) color(red)(cancel(color(black)("mol dm"^(-3)"s"^(-1)))))/(11.0 * color(red)(cancel(color(black)(10^(-8))))color(red)(cancel(color(black)("mol dm"^(-3)"s"^(-1))))) = color(red)(cancel(color(black)(k)))/color(red)(cancel(color(black)(k))) * (0.20)^n/(0.40)^n color(red)(cancel(color(black)(("mol dm"^(-3))^n)))/color(red)(cancel(color(black)(("mol dm"^(-3))^n)))`

`2.75/11.0 = (0.20/0.40)^n`

This is equivalent to

`ln(2.75/11.0) = ln[ (0.20/0.40)^n]`

Solve for `n` to get

`ln(1/4) = n * ln(1/2)`

`ln[(1/2)^2] = n * ln(1/2)`

`2 * color(red)(cancel(color(black)(ln(1/2)))) = n * color(red)(cancel(color(black)(ln(1/2))))`

Therefore,

`n = 2`

and your decomposition reaction is second-order.

SIDE NOTE The order of the reaction must come out the same regardless of what data you chose to use in the calculations.

I recommend using all the possible combinations of data sets to see that this is the case.

`ln(24.75/11.0) = n * ln(0.60/0.40)`

`ln(2.75/24.75) = n * ln(0.20/0.60)`