A parallelogram has sides A, B, C, and D. Sides A and B have a length of #5 # and sides C and D have a length of # 8 #. If the angle between sides A and C is #(5 pi)/8 #, what is the area of the parallelogram?
1 Answer
Explanation:
So let's sketch the scenario. Remember that in a parallelogram, opposite angles are congruent and adjacent angles are supplementary.
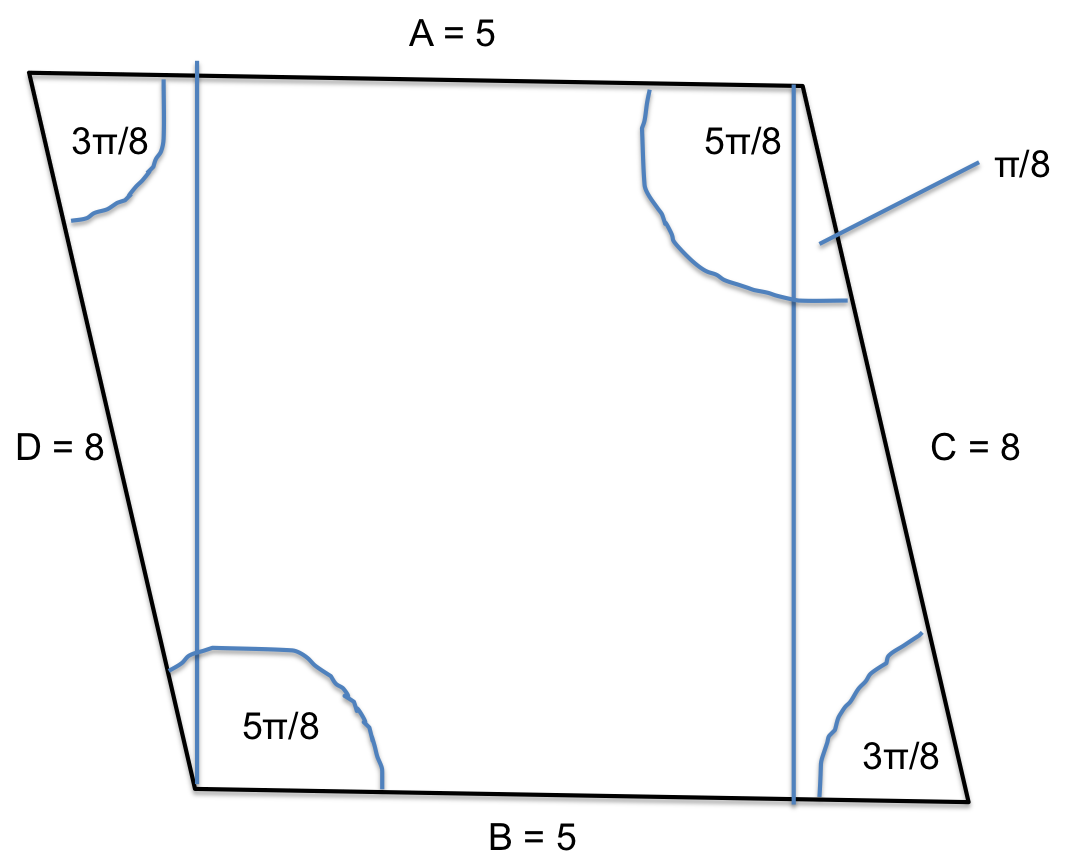
To find the area, we will split the shape into three shapes. Two right angled triangles and a rectangle.
We find the length of the vertical lines (the height of the triangles) by trigonometry. Remember SOH CAH TOA, so sine is opposite/hypotenuse.
We find the base of the triangles with the same technique:
The area of each triangle is given by
If we use
The area outside the rectangle is given by
The rectangle's dimensions are given by the height of the triangles and
As a sense check, if the shape was just a rectangle the area would be 40 so this is in the right neck of the woods at least.

