A triangle has sides A, B, and C. The angle between sides A and B is #(pi)/4#. If side C has a length of #9 # and the angle between sides B and C is #( 3 pi)/8#, what are the lengths of sides A and B?

Question

1435 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-05-24T15:14:23

#A=B=11.791# to 3 decimal places

Particularly so in geometry it is a good idea to do a rough sketch so that you can see what is happening:

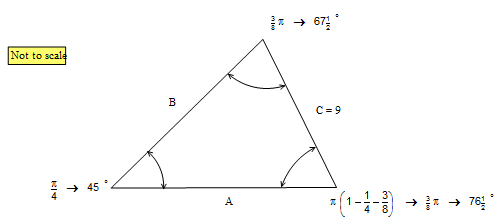
Tony B

Known: Sum of the internal angles of a triangle is 180 degrees

#=> /_AC = pi( 1-1/4 - 3/8) = (3pi)/8 -> 67 1/2 color(white)()^0#

Using the sine rule #->9/(sin(pi/4))=B/(sin((3pi)/8))= A/(sin((3pi)/8 ))#

#=> A=B = (9sin((3pi)/8))/(sin(pi/4))=11.791# to 3 decimal places