An object with a mass of #12 kg# is lying still on a surface and is compressing a horizontal spring by #1/3 m#. If the spring's constant is #6 (kg)/s^2#, what is the minimum value of the surface's coefficient of static friction?
1 Answer
Aug 16, 2017
Explanation:
We can use Newton's second law to solve.
Diagram:
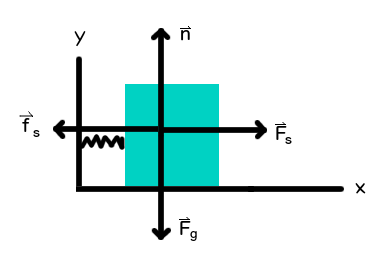
We have the following information:
#|->m=12" kg"# #|->Deltas=-1//3" m"# #|->k=6" kg"//"s"^2# #|->g=9.81"m"//"s"^2#
We can sum the forces parallel and perpendicular:
#sumF_x=F_s-f_s=0#
#sumF_y=n-F_g=0#
- Note that the net force in both directions is 0 as the object remains at rest. The system is in a state of static equilibrium.
We also know:
-
#f_(s"max")=mu_sn=>f_s<=mu_sn# -
#F_s=-kDeltas# -
#F_g=mg#
Substituting the above equations into the statements we formed above:
-
#n=mg# -
#-kDeltas-mu_(s"max ")mg=0#
We can solve for
#color(darkblue)(mu_(s"max ")=(-kDeltas)/(mg))#
Submitting in our known values:
#mu_(s"max ")=-((6" kg"//"s"^2)(-1//3" m"))/((12" kg")(9.81"m"//"s"^2)#
#=>~~0.017#
Therefore,

