Question

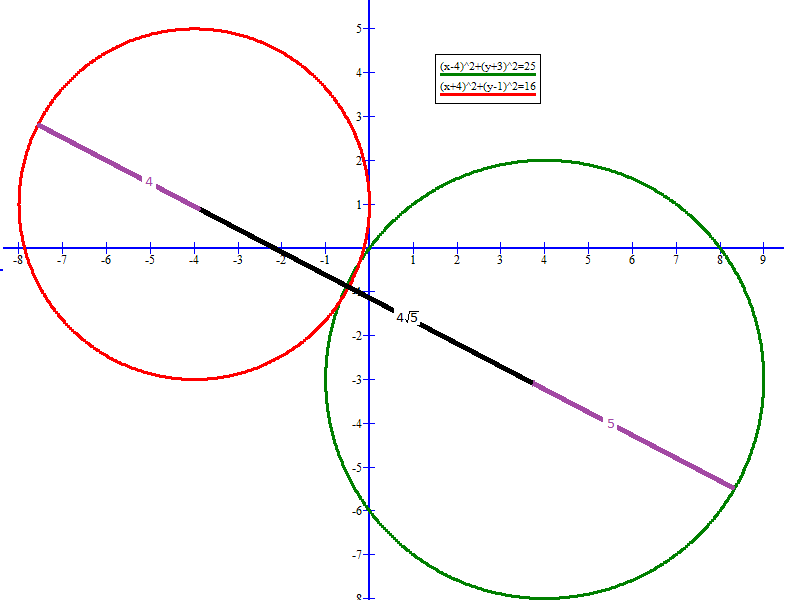
Circles A and B have the following equations `(x -4 )^2+(y +3 )^2= 25` and `(x +4 )^2+(y -1 )^2= 16`. What is the greatest possible distance between a point on circle A and another point on circle B?

Answer 1

`color(green)(9+4sqrt(5))`

Explanation:

The greatest possible distance will be along the extended line joining the centers of the two circles, between the farthest points of each circle from the other.

Circle A:
`(x-4)^2+(y+3)^2=25`
`color(white)("XXX")`has its center at `(4,-3)`
`color(white)("XXX")`and a radius of `5`

Circle B
`(x+4)^2+(y-1)^2=16`
`color(white)("XXX")`has its center at `(-4,1)`
`color(white)("XXX")`and a radius of `4`

The distance between the two centers is
`color(white)("XXX")sqrt((4-(-4))^2+(-3-1)^2)=sqrt(8^2+4^2)=4sqrt(5)`

The greatest distance between two points on these circles would be
`color(white)("XXX")5+4sqrt(5)+4 = 9+4sqrt(5)`