How do you evaluate # cos(arctan(2) +arctan(3))#?
2 Answers
Apr 16, 2016
Explanation:
Consider the following two right angled triangles:
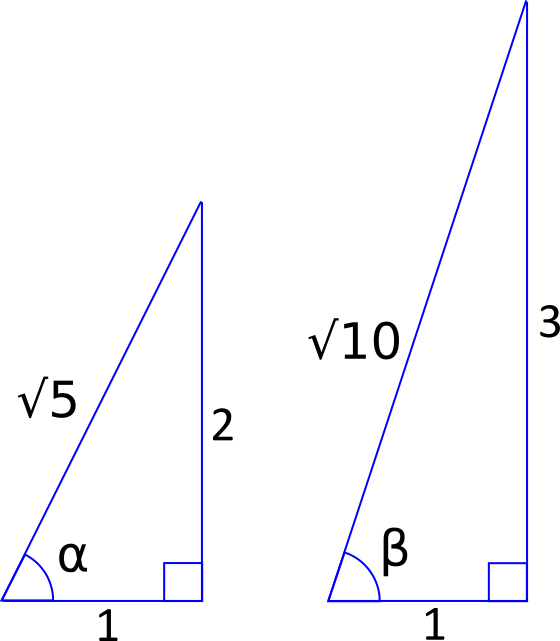
Then:
#{ (tan(alpha) = 2), (tan(beta) = 3) :}#
Notice that:
#{ (sin(alpha) = 2/sqrt(5)), (cos(alpha) = 1/sqrt(5)), (sin(beta) = 3/sqrt(10)), (cos(beta) = 1/sqrt(10)) :}#
The sum of angles formula for
#cos(alpha+beta) = cos(alpha) cos(beta) - sin(alpha) sin(beta)#
#=1/sqrt(5) 1/sqrt(10) - 2/sqrt(5) 3/sqrt(10)#
#=(1-6)/sqrt(50)=-5/(5sqrt(2))=-1/sqrt(2)=-sqrt(2)/2#
Jul 16, 2016


