Question

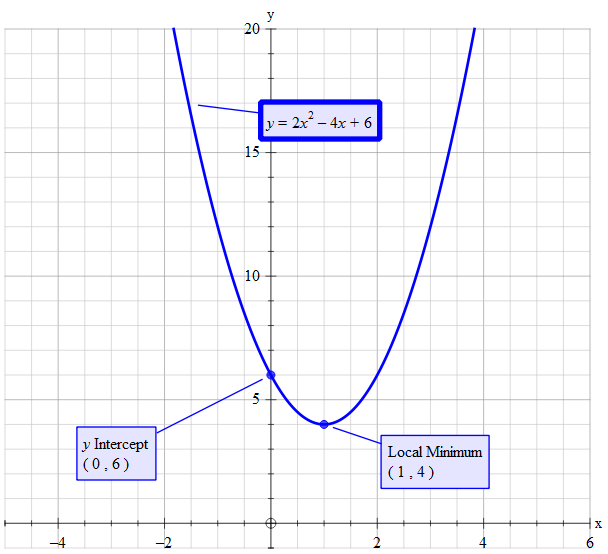
How do you find the vertex of `f(x)= 2x^2-4x+6`?

Answer 1

Vertex`->(x,y)=(1,4)`

Explanation:

Using part of the process of completing the square to determine the value of `x`. Then determining the value of `y` by substitution.

Given:`" "y=2x^2-4x+6`

Write as:`" "y=2(x^2color(red)(-4/2)x)+6`

`x_("vertex")=(-1/2)xx(color(red)(-4/2))= 1`

By substitution:

`y_("vertex")=2(1)^2-4(1)+6 = 4`

Vertex`->(x,y)=(1,4)`

Compare to the standardised form of `y=ax^2+bx+c`

Notice that in the graph that `y_("intercept") = c=6`