Question

How do you graph `y = 3/2 cot( pi/2) x`?

Answer 1

it is a strait line graph with a very steep positive gradient.

Did you mean `y = 3/2 cot[ (pi/2)x] = 3/2 cot((pix)/2)` ?

Explanation:

If you did mean that it should be `cot((pix)/2)` then this is a very different question!!!
graph of `y = 3/2 times cot((pix)/2)`

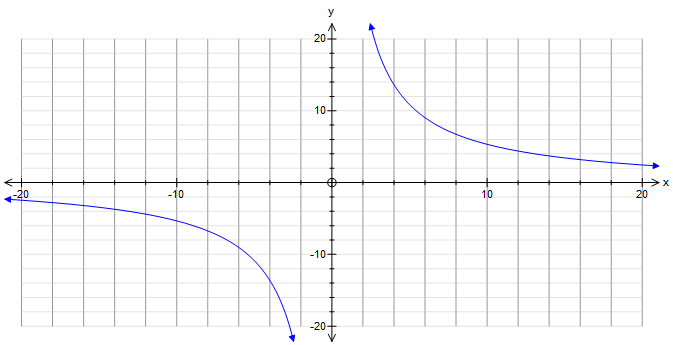
Consider the presented question of `y= 3/2 times cot(pi/2) times x`

`3/2` is a constant

`pi/2` is a constant so `cot(pi/2)` is also a constant

The result is that `x` is multiplied by some constant.

The thing is, are you measuring the angle in degrees or radians?

Note: cot = `1/tan` so in effect you have:

`y = 3/2 times x/(tan(pi/2)) = (3x)/(2 tan(pi/2))`

which may also be written as:

`y = 3/(2 tan(pi/2))" " x`

If just degrees then you would be looking at:

`y ~= 3/(2 times 0.055...) x`

`y ~= 54.7 x` to 1 decimal place.

If on the other hand you are measuring in radians and we have say `(pi " radians")/2` then it is `(180^0)/2 -> tan(90^0)` which is a problem!!!