How do you simplify #sqrt735/sqrt5#?

Question

3636 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-06-30T12:36:37

#sqrt735/sqrt5=7sqrt3#

#sqrt735/sqrt5#

= #sqrt(3xx5xx7xx7)/sqrt5#

= #sqrt((3xx5xx7xx7)/5)#

= #sqrt((3xxcancel5xx7xx7)/cancel5)#

= #sqrt(3xxul(7xx7))#

= #7sqrt3#

Answer 2 · 2016-07-04T10:59:48

#7sqrt(3)#

#color(blue)("Method")#

We need to look for integer factors of 735 and with a bit of luck be able to cancel out the #sqrt(5)# denominator.

Suppose we had 2 unknown variables #a" and "b#. Suppose these 2 variables were presented in the form of

#sqrt(a)/sqrt(b)# This we can write as #sqrt(a/b)#
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
#color(blue)("Answering your question")#

Notice that the sum of the digits in 735 is 15. As 15 is divisible by 3 then 735 is also divisible by 3
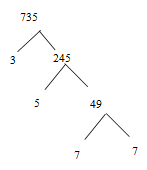
Tony B

From the factor tree we observe that 735 is the product of #3xx5xx7^2#

Write #sqrt(735)/sqrt(5)# as #sqrt((3xxcancel(5)xx7^2)/cancel(5))#

We can take the #7^2# outside the square root but it becomes just #7# giving:

#7sqrt(3)#