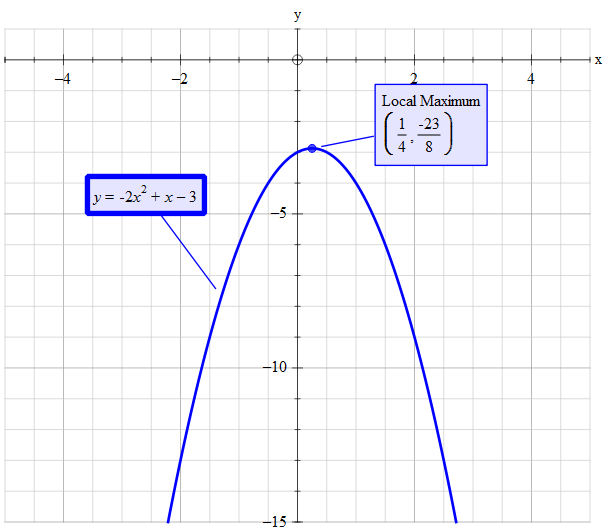
How many points does #y=-2x^2+x-3# have in common with the vertex and where is the vertex in relation to the x axis?
1 Answer
Jun 8, 2017
The number of points in common with the vertex and the graph is 1
Explanation:
This is a quadratic equation and the coefficient of
The vertex has only one point.
Not really a cheat as it is part of the process for completing the square.
Write as:
Note that