Three towns (A,B, and C) are located so that B is 25 km from A and C is 34 km from A. If <ABC is 110 degrees, how do you calculate the distance from B to C?

Question

9792 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2015-09-07T14:59:25

#x=(34sin (70 - sin^-1((25sin110)/34)))/sin110#

First, let's draw the triangle in question using all the given.

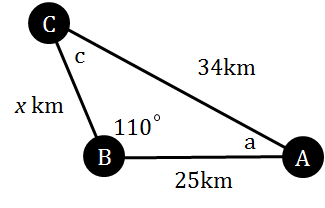
Not to scale.
In this diagram, #a# and #c# are angles, and #x# is a side length.

By the Law of Sines, we know that:

#sin a/x = sin110/34 = sinc/25#

We can immediately solve for #c#.

#sin110/34 = sinc/25#

#(25sin110)/34=sinc#

#c=sin^-1((25sin110)/34)#

The sum of angles in a triangle is #180#, so we can solve for a:

#180 = a + 110 + sin^-1((25sin110)/34)#

#a = 70 - sin^-1((25sin110)/34)#

We now just need to solve the following equation for #x#:

#sin (70 - sin^-1((25sin110)/34))/x = sin110/34#

#(34sin (70 - sin^-1((25sin110)/34)))/sin110 = x#

None of the angles or values used are "standard" values on the unit circle, so this is the final answer.