What is the relationship between the rectangular form of complex numbers and their corresponding polar form?
1 Answer
The rectangular form of a complex form is given in terms of 2 real numbers a and b in the form: z=a+jb
The polar form of the same number is given in terms of a magnitude r (or length) and argument q (or angle) in the form: z=r|_q
You can "see" a complex number on a drawing in this way:
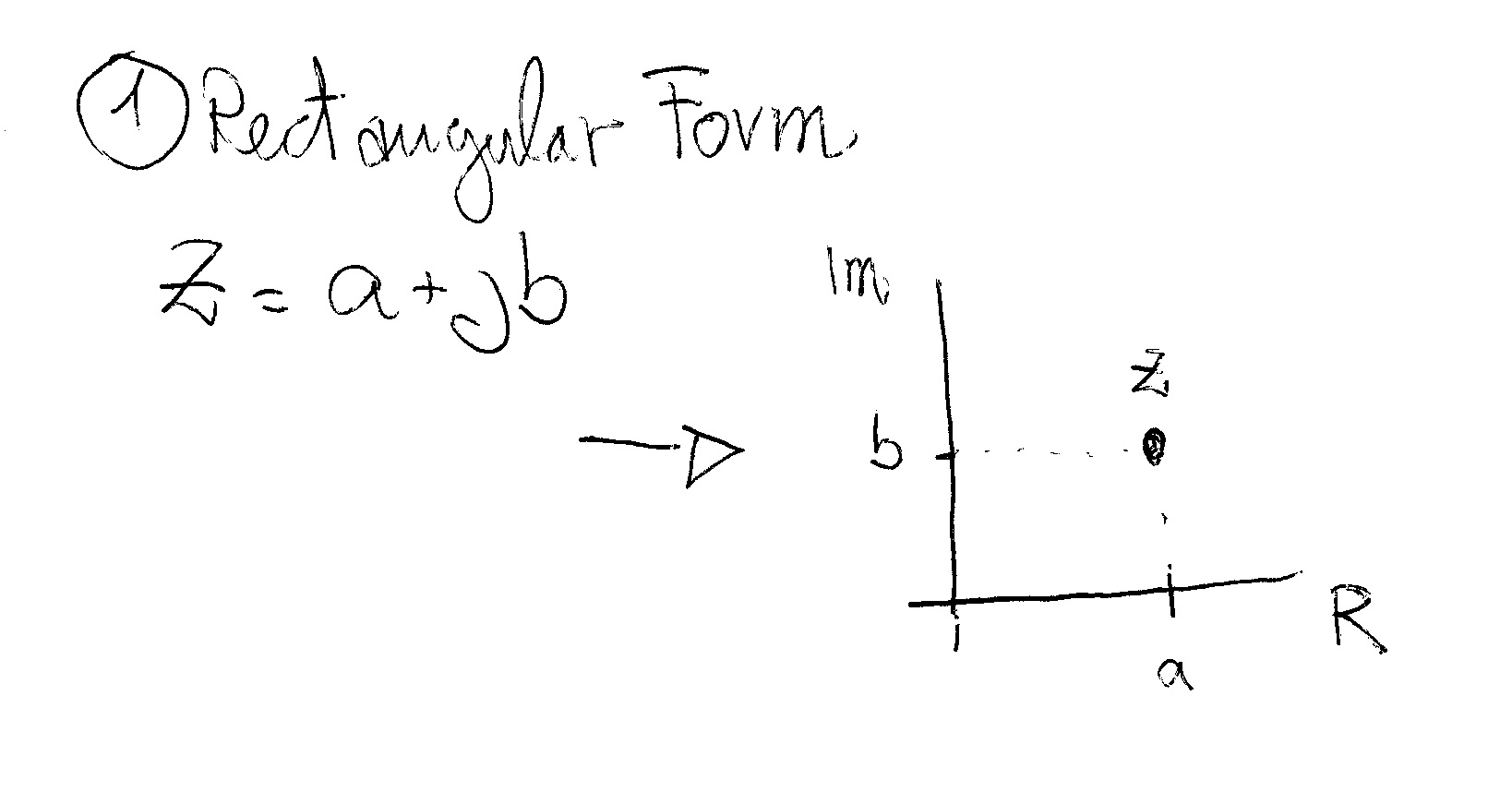
In this case the numbers a and b become the coordinates of a point representing the complex number in the special plane (Argand-Gauss) where on the x axis you plot the real part (the number a) and on the y axis the imaginary (the b number, associated with j).
In polar form you find the same point but using the magnitude r and argument q:
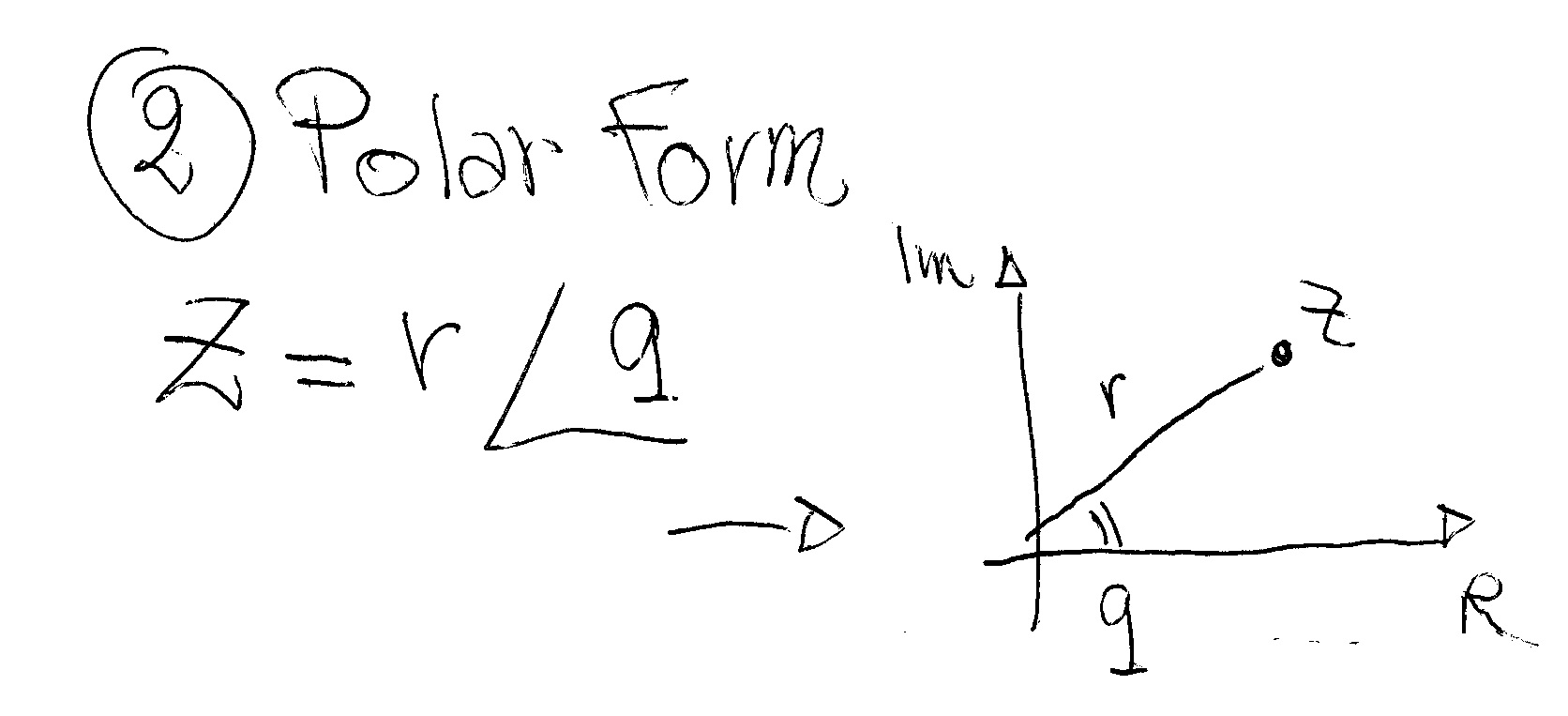
Now the relationship between rectangular and polar is found joining the 2 graphical representations and considering the triangle obtained:
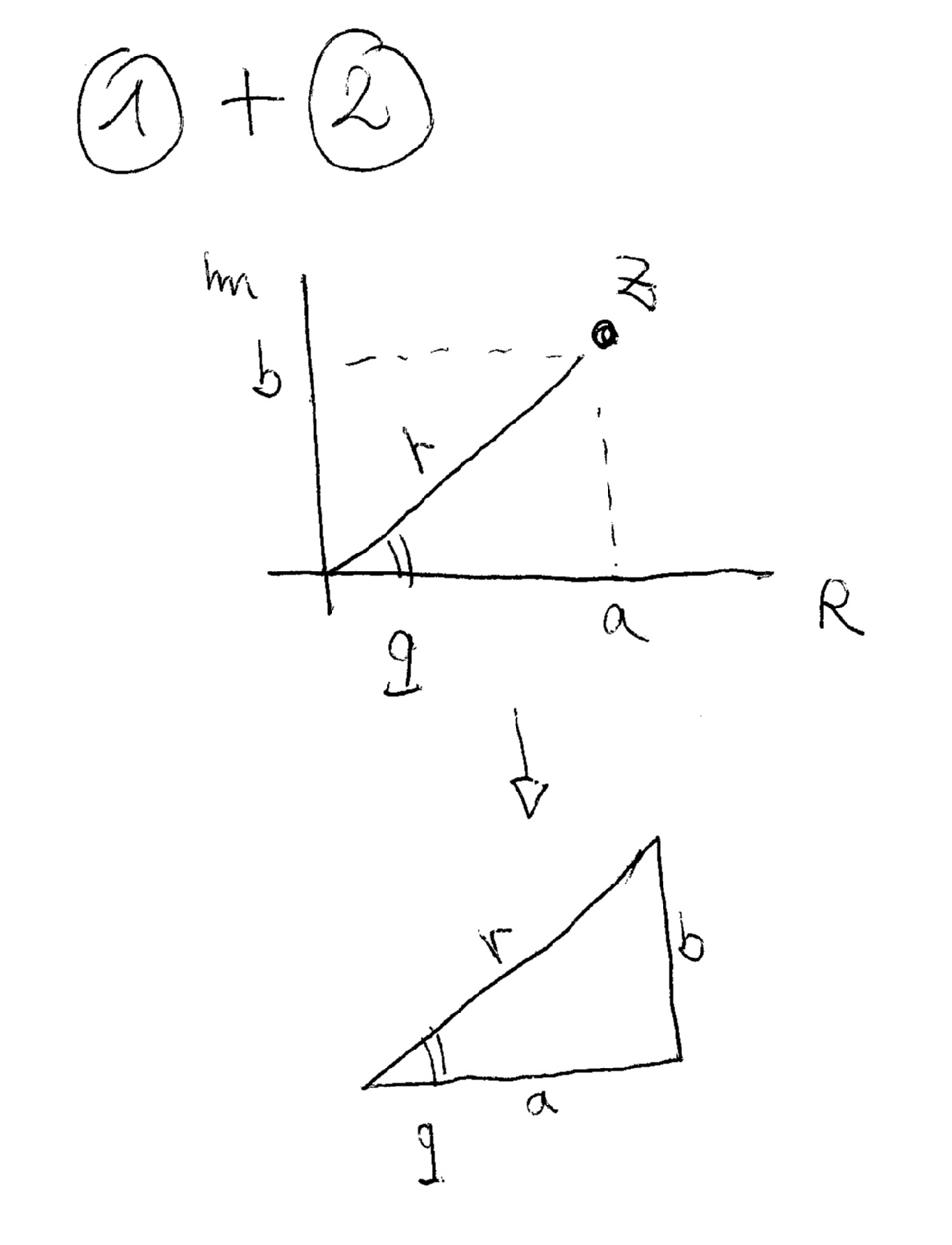
The relationships then are:
1) Pitagora's Theorem (to link the length r with a and b):
2) Inverse trigonometric functions (to link the angle q with a and b):
I suggest to try various complex numbers (in diferente quadrants) to see how these relationships work.

