Question

What is the graph of `f(x) = x^2`?

Answer 1

The graph of `f(x)=x^2` is called a "Parabola." It looks like this:

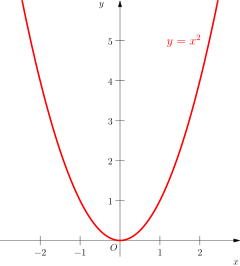
One of the ways to graph this is to use plug in a few x-values and get an idea of the shape. Since the x values keep getting squared, there is an exponential increase on either side of the y-axis. You can see this by plugging in a few values:

When
`x=0, f(x)=0`
`x=1, f(x)=1^2=1`
`x=2,f(x)=2^2=4`
`x=3, f(x)=3^2=9`
`x=4, f(x)=4^2=16`

The same holds true for negative x-values to the left of the y-axis since a negative value squared is positive. For example,

`x=-1, f(x)=(-1)^2=-1*-1=1`
`x=2,f(x)=(-2)^2=-2*-2=4`