Question

What is the antiderivative of `xsqrtx`?

Answer 1

You can simply multiply them together (more explicitly).

`xsqrtx = x^("3/2")`

And then just use the reverse Power Rule.

`d/(dx)[x^("3/2")] = 2/5x^("5/2")`

Then, since an antiderivative is a generalization of what an integral does, they are almost the same thing. Therefore, we add a constant to imply that you get every single function that is within the antiderivative's slope field.

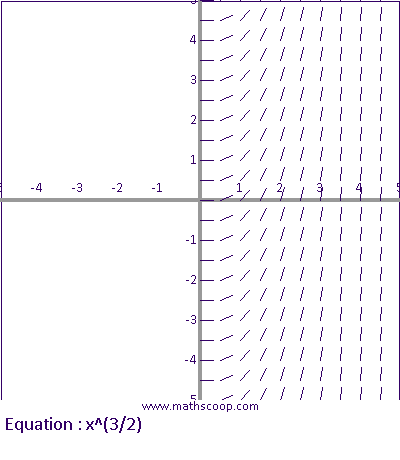
(notice the various vertical-shift variations of a single function forms the slope field)

`-> color(blue)(2/5x^("5/2") + C)`

Answer 2

`2/5 x^(5/2)`

Explanation:

Note : `x sqrt(x) = x x^(1/2) = x^(1 + 1/2) = x^(3/2)`
Therefore, `int x sqrt(x) dx = int x^(3/2) dx = (x^(3/2 + 1)) / (3/2 + 1) = 2/5 x^(5/2)`