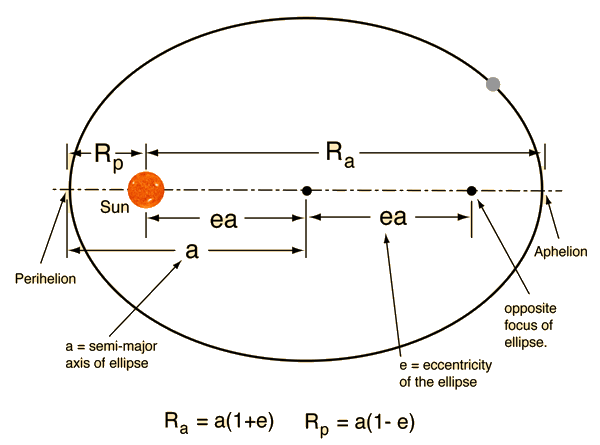
The semi-major axis, #a# of and ellipse is half of the major axis, the total distance between perihelion and aphelion. The semi-major axis is therefore equal to;
#a = (R_a + R_p)/2 = (6.0"AU"+2.0"AU")/2 = 4.0"AU"#
Eccentricity is defined as the ratio of the distance between two focus of the ellipse, #R_a - R_p#, and the length of the major axis, #R_a + R_p#. This can be expressed mathematically as;
#e = (R_a - R_p)/(R_a + R_p) = (6.0"AU" - 2.0"AU")/(6.0"AU"+2.0"AU")=.5#
The period, #T#, can be found using the Kepler's 3rd law, which states that the ratio of the period squared to the semi-major axis cubed is a constant for all objects orbiting the same body. In other words, for two objects that orbit the sun;
#T_1^2/a_1^3 = T_2^2/a_2^3 = C#
Or, restated;
#T_1^2 = a_1^3/a_2^3 T_2^2#
If we use the Earth as object #2#, then #T_2# and #a_2# are both #1#. We can calculate the period of the asteroid using its semi-major axis, #a=4.0"AU"#.
#T_1^2 = (4.0"AU")^3/(1"AU")^3 (1"year")^2 = 4.0"years"^2#
Taking the square root, we get a period of #2"years"# for the asteroid.


