Two corners of an isosceles triangle are at (8 ,1 ) and (1 ,7 ). If the triangle's area is 15 , what are the lengths of the triangle's sides?
1 Answer
Two possibilities: (I)
Explanation:
The length of the given side is
From the formula of the triangle's area:
Since the figure is an isosceles triangle we could have Case 1 , where the base is the singular side, ilustrated by Fig. (a) below
 I created this figure using MS Excel
I created this figure using MS Excel
Or we could have Case 2 , where the base is one of the equal sides, ilustrated by Figs. (b) and (c) below
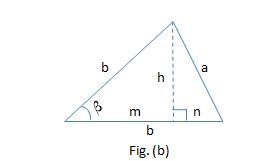 I created this figure using MS Excel
I created this figure using MS Excel
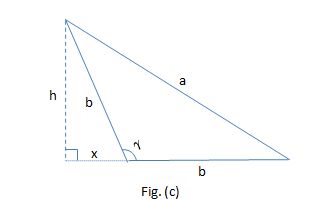 I created this figure using MS Excel
I created this figure using MS Excel
For this problem Case 1 always applies, because:
tan(alpha/2)=(a/2)/h =>h=(1/2)a/tan(alpha/2)
But there's a condition so that Case 2 apllies:
sin(beta)=h/b =>h=bsin beta
Orh=bsin gamma
Since the highest value ofsin beta orsin gamma is1 , the highest value ofh , in Case 2, must beb .
In the present problem h is smaller than the side to which it is perpendicular, so for this problem besides the Case 1, also the Case 2 applies.
Solution considering Case 1 (Fig. (a)),
b^2=h^2+(a/2)^2
b^2=(30/sqrt(85))^2+(sqrt(85)/2)^2
b^2=900/85+85/4=180/17+85/4=(720+1445)/68=2165/68 =>b=sqrt(2165/68)~=5.643
Solution considering Case 2 (shape of Fig. (b)),
b^2=m^2+h^2
m^2=b^2-h^2=(sqrt(85))^2-(30/sqrt(85))^2=85-900/85=85-180/17=(1445-180)/17 =>m=sqrt(1265/17)
m+n=b =>n=b-m =>n=sqrt(85)-sqrt(1265/17)
a^2=h^2+n^2=(30/sqrt(85))^2+(sqrt(85)-sqrt(1265/17))^2
a^2=900/85+85+1265/17-2sqrt((85*1265)/17)
a^2=180/17+85+1265/17-2*sqrt(5*1265)
a^2=1445/17+85-2*5sqrt(253)
a^2=85+85-10sqrt(253)
a=sqrt(170-10sqrt(253))~=3.308

