Question

What is a parsec?

Answer 1

A unit of length.

Explanation:

It's definition is a little hard to understand, but it is the distance where 1 Astronomical Unit (AU) subtends an angle of 1 arcsecond (or `1/3600` of a degree). It is equal to 3.26156 light-years.

See the picture below for a visual.

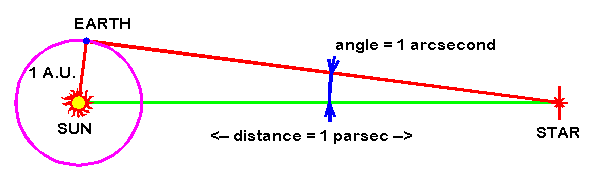
Let's do the calculations. Let `R` be the distance of a star 1 parsec away and `r=1AU` be the radius of the Earth's orbit and `theta` be the parallax angle which is 1 arc second by definition. As the angle is small we can use the formula `r=R theta`, where `theta` is in radians to relate the values.

Converting `theta` to radians gives:
`theta = 1/(360*60*60) * pi/180`
So, we can calculate the value of a parsec `R` in AU as:
`R=r/theta=1/theta=(360*60*60*180)/pi=206,264.8AU`
Now `1UA=1.58125*10^(-5)` light years.
We ccan now calculate the value of a parsec in light years as:
`R=206,264.8*1.58125*10^(-5)=3.26156` light years.