How do you find the area between f(x)=x+1 and g(x)=(x-1)^2?
1 Answer
The area is
Explanation:
Start by finding the intersection points of the curves.
{(y = x+ 1), (y = (x - 1)^2):}
Solve by substitution.
x + 1= (x- 1)^2
x+ 1 = x^2 - 2x + 1
0 = x^2 - 3x
0 = x(x - 3)
x= 0 and 3
We now draw the two curves to see which one is above and which one is below. This is important, as we will then find the area under the higher curve and subtract the area of the lower curve.
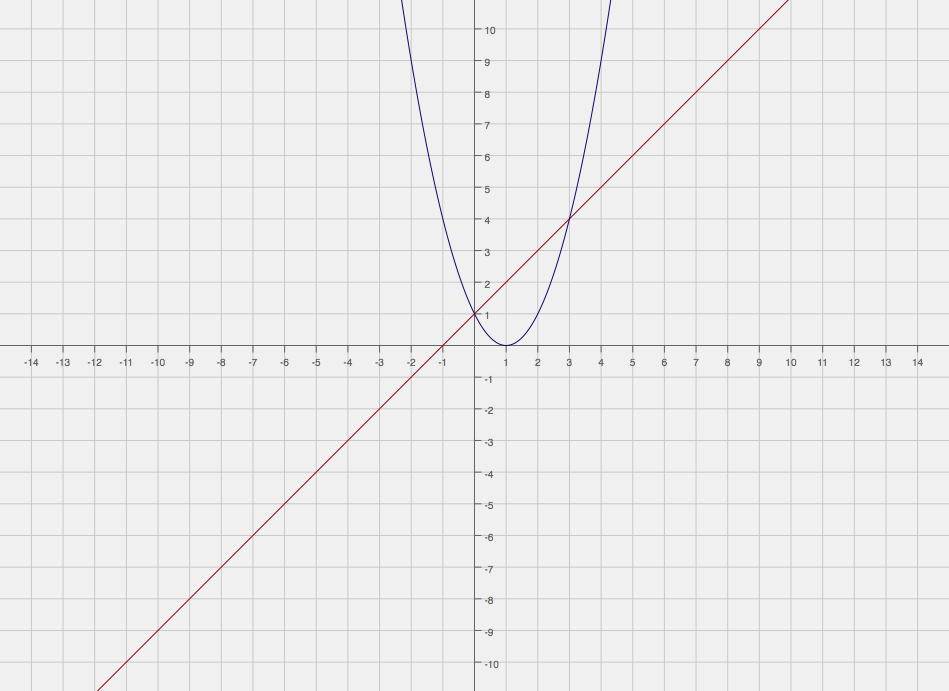
So, we find the area of the linear function (
Hopefully this helps!

