Question

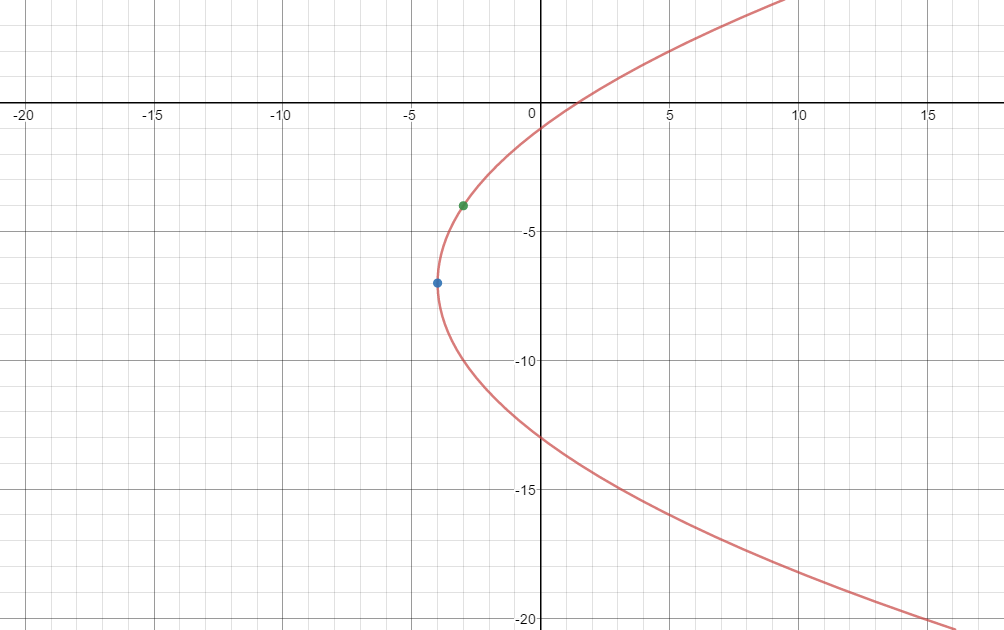
How do you write the equation of the parabola in vertex form given vertex (-4,-7) and also passes the point (-3,-4)?

Answer 1

There are 2 equations, one of the form, `y=a(x - h)^2+k`, and the other of the form, `x=a(y-k)^2+h`. Where `(h,k)` is the vertex and you solve for "a" using the given point.

Explanation:

Given the vertex `(-4,-7)` and passes through the point `(-3,-4)`

Using the first form:

`y = a(x- -4)^2 -7`

Substitute -3 for x and -4 for y:

`-4 = a(-3- -4)^2 -7`

`3 = a(1)^2`

`a = 3`

The first equation is:

`y = 3(x- -4)^2 - 7`

Here is the graph of the equation and the two points:

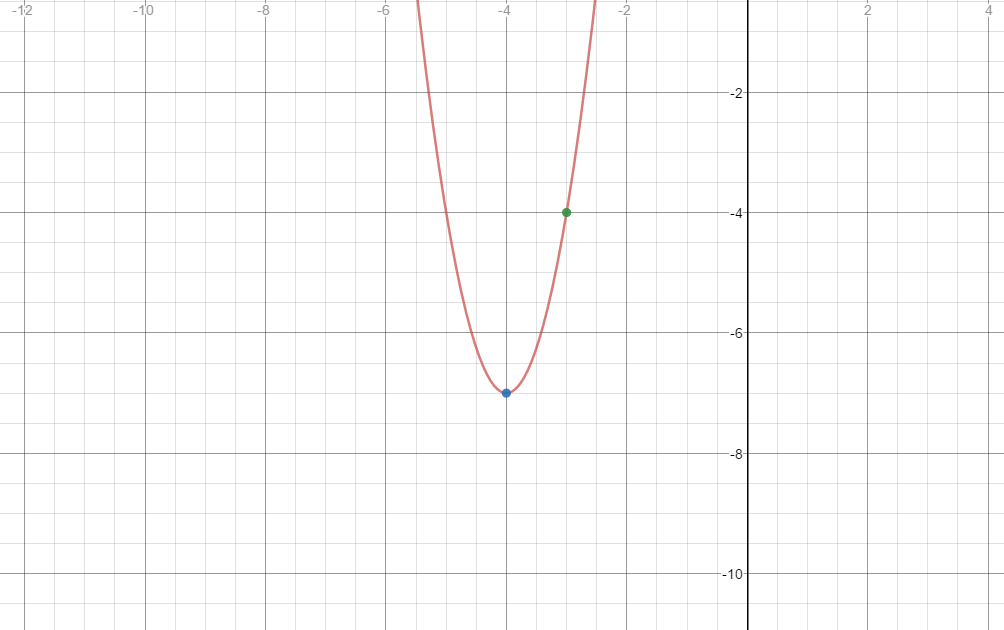
Using the second form:

`x = a(y- -7)^2-4`

Substitute -3 for x and -4 for y:

`-3 = a(-4- -7)^2 -4`

`1 = a(3)^2`

`a = 1/9`

The second equation is:

`x = 1/9(y- -7)^2 - 4`

Here is the graph of the equation and the two points: