Question

What is the equation of the line with slope `m= -11/5` that passes through `(-13/15,-13/24)`?

Answer 1

See below.

Explanation:

Based on the given information, you can use the point-slope form equation to get the desired equations.

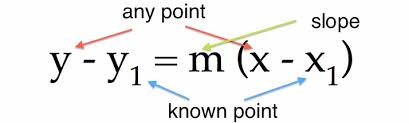
In this case, you would plug in `m = - (11/5)` for `m` in the point-slope form, along with the x- and y-coordinates of `(-13/15 , -13/24)` for `x1` and `y1` in the equation. Then, you would get this:

`y - (-13/24) = (-11/5)(x - (-13/15))`.

This can be simplified to:

`y + 13/24 = -11/5(x+13/15)`.

This would be your final answer, unless your instructor wants you to express the final answer in slope-intercept form, which is `y = mx + b`. I am not going to take the extra step since you have not specified what form the equation should be expressed in, but this would be your answer for the problem.

I hope that helps!