Question

What is the electron configuration for the f block?

Answer 1

The f block has the lower level filled, then for valance electrons has 2 s electrons 1 d electron and then up to 14 f electrons filling the 7 f orbitals.

Answer 2

I finally feel confident enough to post a table of the configurations, along with some detailed rationale for why the configurations are so riddled with 'Aufbau exceptions'.

For reference, the energy scales I will be using are small. For perspective, you can compare the numbers with the first ionization energy of `"N"` atom of `"14.53 eV"`, and the first ionization energy of `"H"` atom of `"13.61 eV"`.

The following graphs are from page `199 - 202` of this book by my advisor, as well as Michael Dolg and Kenneth Dyall. All energies here are in hartrees (`E_h`), where `1` `E_h = "27.2114 eV"`.

---

DISCLAIMER: LONG ANSWER!

LANTHANIDES

The order by atomic number is down the first column, and then down the second column. In `color(red)("red")` are the 'Aufbau exceptions'.

`color(white)([(color(red)(La),(color(red)([Xe] 6s^2 5d^1)),color(black)(Tb),(color(black)([Xe] 6s^2 4f^9))),(color(red)(Ce),(color(red)([Xe] 6s^2 4f^1 5d^1)),color(black)(Dy),(color(black)([Xe] 6s^2 4f^10))),(color(black)(Pr),(color(black)([Xe] 6s^2 4f^3)),color(black)(Ho),(color(black)([Xe] 6s^2 4f^11))),(color(black)(Nd),(color(black)([Xe] 6s^2 4f^4)),color(black)(Er),(color(black)([Xe] 6s^2 4f^12))),(color(black)(Pm),(color(black)([Xe] 6s^2 4f^5)),color(black)(Tm),(color(black)([Xe] 6s^2 4f^13))),(color(black)(Sm),(color(black)([Xe] 6s^2 4f^6)),color(black)(Yb),(color(black)([Xe] 6s^2 4f^14))),(color(black)(Eu),(color(black)([Xe] 6s^2 4f^7)),color(black)(Lu),(color(black)([Xe] 6s^2 4f^14 5d^1))),(color(red)(Gd),(color(red)([Xe] 6s^2 4f^7 5d^1)),"","")])`

The exceptions can be explained by looking at how the energies of the `6s`, `5d`, and `4f` orbitals vary for the lanthanides.

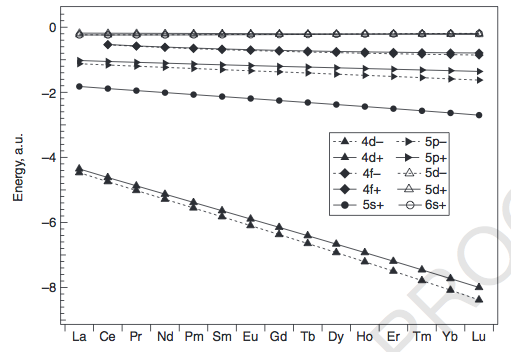
We can see that the `4f` orbitals decrease in energy as we go from left to right, but the `6s` and `5d` orbitals are consistently within `0.1` `E_h` (about `"2.7 eV"`) of each other.

The radii of the `(n-2)f` orbitals are also more contracted, particularly for the lanthanides, making them more core-like in size than even the `5s` and `5p` orbitals, in addition to the decreasing `4f` energies.

The exceptions occur mainly for the earlier lanthanides (`La, Ce`), where the `4f`'s are still fairly close in energy to the `5d` and `6s`.

• The radial compactness of the `4f` orbitals makes it more favorable to fill the `6s` and `5d` first for `La` and `Ce`, to minimize electron repulsion.

• For `Gd`, the repulsion that would be generated from pairing a `4f` electron would be enough to promote it to a `5d` orbital (about `0.6` `E_h` away, or about `"16 eV"`), so `Gd` takes on a `f^7 d^1` configuration instead of `f^8 d^0`.

ACTINIDES

The order by atomic number is down the first column, and then down the second column. In `color(red)("red")` are the 'Aufbau exceptions'.

`color(white)([(color(red)(Ac),(color(red)([Rn] 7s^2 6d^1)),color(black)(Bk),(color(black)([Rn] 7s^2 5f^9))),(color(red)(Th),(color(red)([Rn] 7s^2 6d^2)),color(black)(Cf),(color(black)([Rn] 7s^2 5f^10))),(color(red)(Pa),(color(red)([Rn] 7s^2 5f^2 6d^1)),color(black)(Es),(color(black)([Rn] 7s^2 5f^11))),(color(red)(U),(color(red)([Rn] 7s^2 5f^3 6d^1)),color(black)(Fm),(color(black)([Rn] 7s^2 5f^12))),(color(red)(Np),(color(red)([Rn] 7s^2 5f^4 6d^1)),color(black)(Md),(color(black)([Rn] 7s^2 5f^13))),(color(black)(Pu),(color(black)([Rn] 7s^2 5f^6)),color(black)(No),(color(black)([Rn] 7s^2 5f^14))),(color(black)(Am),(color(black)([Rn] 7s^2 5f^7)),color(black)(Lr),(color(black)([Rn] 7s^2 5f^14 6d^1))),(color(red)(Cm),(color(red)([Rn] 7s^2 5f^7 6d^1)),"","")])`

We can again examine the energies (pg. 199):

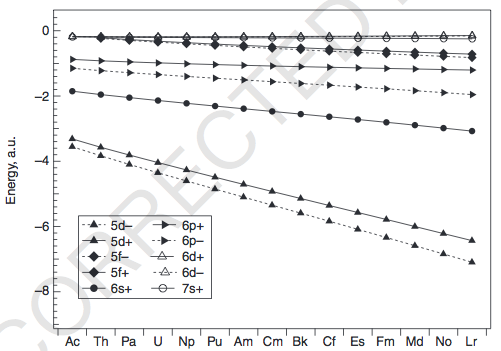
The energies of the `7s` and `6d` are likewise very close to each other (within `"2.7 eV"` as before), but the `5f` are at MOST `0.4` `E_h`, or about `11` `"eV"` away from the `7s` and `6d` orbitals.

That makes the energetic degeneracies of the `5f` with the `6d` and `7s` and the compactness of the `5f` orbitals even more significant in giving rise to `Ac-Np` as 'Aufbau exceptions'.

As before, the exceptions occur mainly for the earlier actinides (`Ac-Np`).

• For `Ac-Th`, since the `5f`'s and `6d`'s are very similar in energy, it is possible for `6d` occupation instead of the `5f`. I believe it is because the `5f` orbitals are barely bigger than the `6p`, `6s`, and `5d` orbitals for `Th` that the `5f` is about as core-like as them and thus not as accessible to fill... but this is difficult to explain.

You can see the radial extents here (pg. 202):

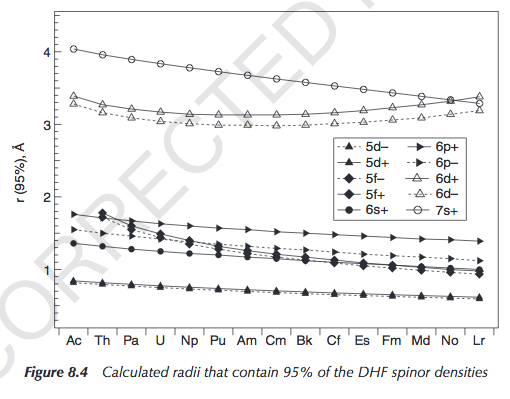
"Spinor" just means an electronic quantum state (in the Pauli Exclusion sense) with a specific spin (up/down). DHF stands for Dirac-Hartree-Fock.

• For `Pa - Np`, whose `6d-5f` gap is even smaller than the `5d-4f` gap of the lanthanides (but bigger than for `Ac-Th`), I believe that the repulsions generated from adding a second electron into the `6d` orbitals (even without pairing) are still enough that since the `5f` orbitals are lower in energy, it is preferable to proceed by filling them instead.

• And again for `Cm`, similar to `Gd`, the electron repulsion that occurs with pairing an `5f` electron would be enough to promote it to a `6d` orbital (about `0.15` `E_h` away, or about `"4 eV"`).