Question

How do you find the area bounded by `y=x^2+2x-4` and `y=-2x^2+4x-3`?

Answer 1

`A= 32/27`

Explanation:

Consider the function:

`f(x) = (x^2+2x-4) - (-2x^2+4x-3)`

`f(x) = 3x^2-2x-1`

The values of `x` for which the two curves intersect are the solutions of the equation:

`f(x) = 0`

`3x^2-2x-1=0`

`x= (1+-sqrt(1+3))/3`

`x_1 = -1/3`, `x_2=1`

Note now that as `f(x)` is a second degree polynomial with leading positive coefficient, its value is negative in the interval between the roots.

The area bounded by the curves is then:

`A = int_(-1/3)^1 abs(f(x))dx = int_(-1/3)^1 (-3x^2+2x+1)dx`

`A = [ -x^3+x^2+x ]_(-1/3)^1`

`A =-1+1+1-1/27-1/9+1/3 = (27-1-3+9)/27 = 32/27`

Answer 2

Use the integral of the difference between the two functions:

`"Area" = int_a^bf(x)-g(x)dx; f(x)>g(x), (a,b)`

Explanation:

Here is a graph of

`y=x^2+2x-4" [1]"` (in red)

and

`y=-2x^2+4x-3" [2]"` (in blue)

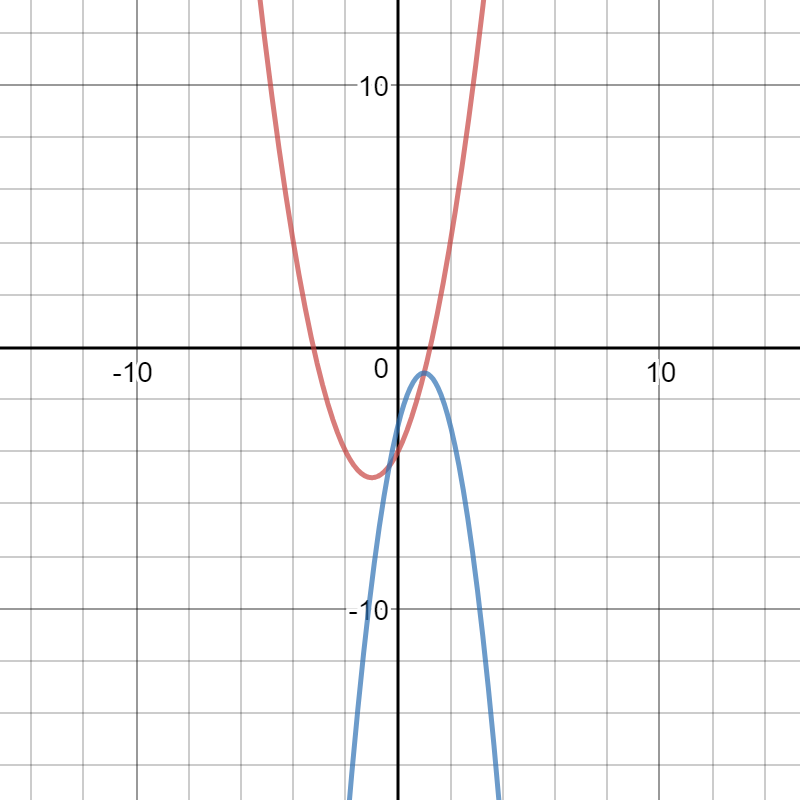
Pleases observe that equation [2] is greater than equation [1] in the enclosed region; this means that the integral is of the form:

`int_a^b -2x^2+4x-3- (x^2+2x-4) dx`

Simplify the integrand:

`int_a^b -3x^2+2x+1 dx`

We need to find the values of "a" and "b" by finding the two x coordinates where the two parabolas intersect. We can do this by setting the integrand equal to 0 and then solving for the two values of x:

`-3x^2+2x+1 = 0`

Multiply by -1:

`3x^2-2x-1 = 0`

This factors into:

`(x-1)(3x+1)`

`x = 1 and x = -1/3`

The above are the values of "a" and "b":

`int_(-1/3)^1 -3x^2+2x+1 dx =`

`[-x^3 + x^2+x]_(-1/3)^1 =`

`-(1)^3+ (1)^2+1 + (1/3)^3-(1/3)^2-1/3 = 32/27`