Question

What is the orthocenter of a triangle with corners at `(4 ,5 )`, `(8 ,3 )`, and (7 ,9 )#?

Answer 1

Coordinates of orthocenter `(56/11, 57/11)`

Explanation:

Orthocenter is the intersection point of the three altitudes of a triangle

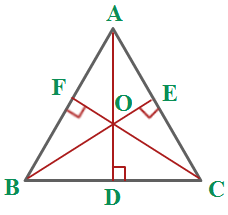
Slope of BC `= m1 = (9-3)/(7-8) = -6`
Slope of (AD) altitude passing through point `A = -1/(m1 )= 1/6`

Eqn of (AD) Altitude through point A is
`(y-5) = (1/6)(x-4)`
`6y - 30 = x - 4`
`x - 6y = -26` Eqn (1)

Slope of AC `= m2 = (9-5)/(7-4) = 4/3`
Slope of (BE) altitude passing through point B `= -1/(m2) = -(3/4)`

Eqn of (BE) Altitude passing through point B is
`(y-3) = -(3/4)(x-8)`
`4y-12 = -3x + 24`
`3x + 4y = 36`. Eqn (2)

Solving Eqns (1), (2) we get the orthocenter coordinates.

`11x = -52 +108 = 56, x = 56/11`
`22y = 114, y = 57/11`

Coordinates of orthocenter (56/11, 57/11)

This can be verified by solving the third altitude passing through point C as all the three altitudes intersect at the orthocenter.