Question

A triangle has sides with lengths: 7, 2, and 14. How do you find the area of the triangle using Heron's formula?

Answer 1

No area

Explanation:

The triangle has no area, and it cannot exist.

The triangle inequality states that,

`a+b>c`

`b+c>a`

`c+a>b`

In words, the sum of a triangle's two sides' lengths is always bigger than the remaining one.

But here, we get: `7+2=9<14`, and so this triangle cannot exist.

Answer 2

`color(brown)("We can not form a triangle with the given measurements."`

Explanation:

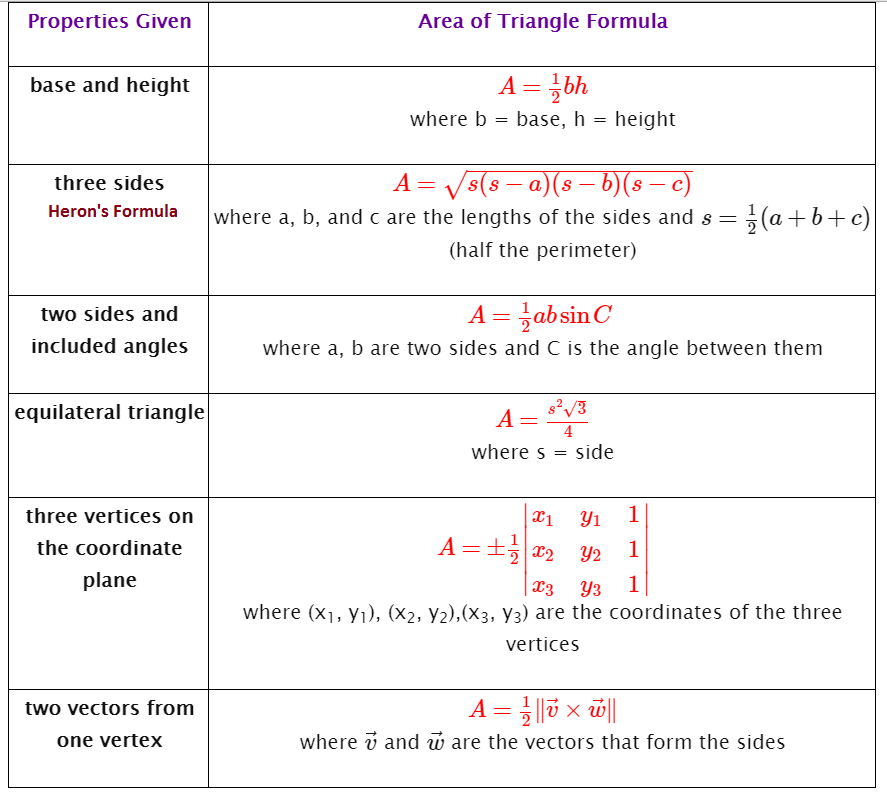
Given : `a = 7, b = 2, c = 14`

To find the area of the triangle using Heron's Formula.

Heron's Formula `A_t = sqrt(s (s-a) (s-b) (s-c)) " , where " s = (a + b + c) / 2`

`s = (7 + 2 + 14) / 2 = 11.5`

`color(red)("For a triangle to exist, sum of any two sides must be greater than the third side"`

In this case, `a + b < c`.

`color(brown)("Hence, we can not form a triangle with the given measurements."`