Two corners of an isosceles triangle are at (2 ,5 ) and (6 ,7 ). If the triangle's area is 4 , what are the lengths of the triangle's sides?
1 Answer
Explanation:
Since our answer doesn't involve a point, let's try to "turn" the triangle and get rid of the points. I assume that the segment between the two point is the base of the triangle, meaning that the other two sides are equal since the triangle is isosceles.
So, how long is the base of this triangle? That's an important part of the equation for the area of a triangle.
Let's use the distance formula to find the distance between these two points. Search it up, you'll use it a lot in geometry.
So how does this factor into the area? This is the base right. half of base times height is the area.
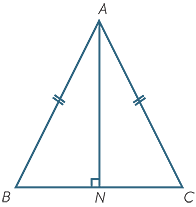
Well, we now know the straight up a height of the triangle. But what is the length of the two equal sides. Draw any isosceles triangle, and draw the height line of it. What angle does a height line in a triangle make with the base by definition? (Hint this angle goes straight up). Well it makes a right angle.
 socratic.org
socratic.org
So this isosceles triangle can be split into two right triangles. For each right triangle, the height of it stays the same. However the base gets halved, so keep track of that.
So we have set up two identical right triangles. We know the length of each leg, but we don't know the length of the hypotenuse (the side opposite the right angle). Use the Pythagorean theorem to figure out the final answer.
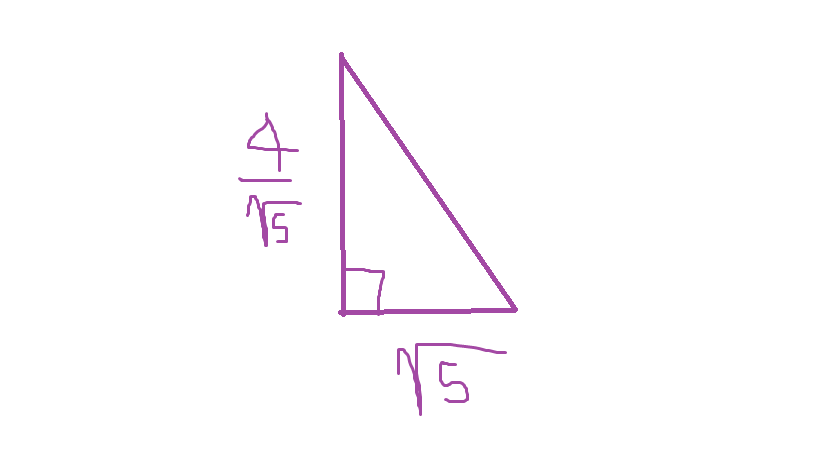 My terrible MS Paint drawing
My terrible MS Paint drawing

