A triangle has corners A, B, and C located at #(4 ,7 )#, #(3 ,5 )#, and #(6 ,2 )#, respectively. What are the endpoints and length of the altitude going through corner C?

Question

1463 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-07-30T10:37:52

The endpoints of altitudes # :N(12/5,19/5) and C(6,2)#
Length of the altitude going through corner C #=sqrt(81/5)~~16.2#

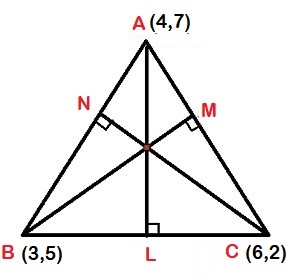
Let #triangleABC " be the triangle with corners at"#

#A(4,7), B(3,5) and C(6,2)#

Let #bar(AL) , bar(BM) and bar(CN)# be the altitudes of sides #bar(BC) ,bar(AC) and bar(AB)# respectively.

Let #(x,y)# be the intersection of three altitudes

enter image source here

Slope of #bar(AB) =(7-5)/(4-3)=2/1=2#

#bar(AB)_|_bar(CN)=>#slope of # bar(CN)=-1/2# ,

# bar(CN)# passes through #C(6,2)#

#:.#The equn. of #bar(CN)# is #:y-2=-1/2(x-6)#

#=>2y-4=-x+6#

#=>x=6-2y+4#

#i.e. color(red)(x=10-2y.....to (1)#

Now, Slope of #bar(AB) =2# and #bar(AB)# passes through
#A(4,7)#

So, eqn. of #bar(AB)# is: #y-7=2(x-4)#

#=>y-7=2x-8#

#=>color(red)(2x-y=1...to(2)#

from #(1)and (2)#

#2(10-2y)-y=1#

#=>20-4y-y=1=>-5y=-19=>color(blue)(y=19/5=3.8#

From #(1) ,#

#x=10-2(19/5)==>color(blue)(x=12/5=2.4#

#=>N(12/5,19/5) and C(6,2)#

Using Distance formula,

#CN=sqrt((12/5-6)^2+(19/5-2)^2)=sqrt(324/25+81/25)#
#:.CN=sqrt(405/25)#

#:.CN=sqrt(81/5)~~16.2#