Question

Cups A and B are cone shaped and have heights of `24 cm` and `23 cm` and openings with radii of `11 cm` and `9 cm`, respectively. If cup B is full and its contents are poured into cup A, will cup A overflow? If not how high will cup A be filled?

Answer 1

`~~20.7cm`

Explanation:

Volume of a cone is given by `1/3pir^2h`, hence

Volume of cone A is `1/3pi11^2*24=8*11^2pi=968pi` and

Volume of cone B is `1/3pi9^2*23=27*23pi=621pi`

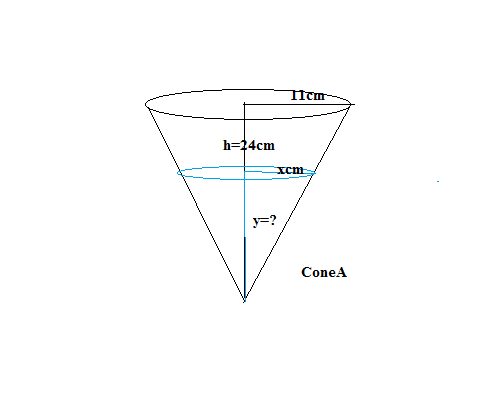
It is obvious that when contents of a full cone B are poured into cone A, it will not overflow. Let it reach where upper circular surface will form a circle of radius `x` and will reach a height of `y`,
then the relation becomes
`x/11=y/24=>x=(11y)/24`
So equating `1/3pix^2y=621pi`
`=>1/3pi((11y)/24)^2y=621pi`
`=>y^3=(621*3*24^2)/11^2~~20.7cm`