How do you evaluate the equation #arctan(pi/2)#?
1 Answer
Nov 11, 2015
Explanation:
There is no "equation" here to evaluate but assuming the question was intended to be
First note that the value
If
we have the situation with opposite to adjacent side ratios as indicated below:
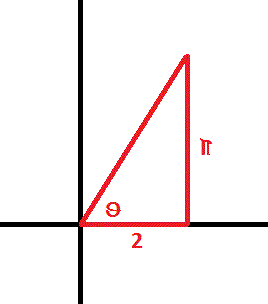
This is not one of the "standard" triangles and the only way to (reasonably) evaluate it is to use a calculator (or trig tables) to get the value indicated in the "Answer" above.
