If you're using a graph to find this limit, the first thing you'll want to do is graph the function.
#f(x)=x^2+2# is a parabola that looks like this:
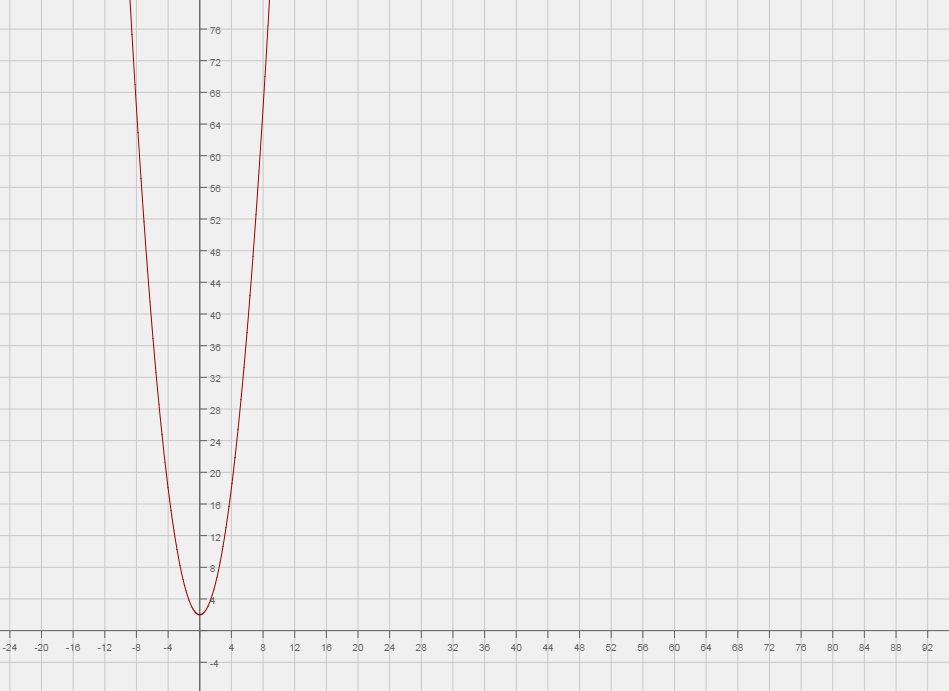
If you want to find out how to graph this, you can either draw the graph of a normal parabola and translate it vertically by two units upwards (2 is being added to the #x^2#, which is why it goes up), or you can create a table of values and plug in input #x# values to get output #y# and you'll get an idea of the shape of the graph.
Now we're interested in knowing what is happening at #x#=5. Luckily, the function is defined there. If we look at the graph, at #x#=5, y=27. It's a little bit hard to tell on the graph because of the exponentially increasing y-values, but we know that #y#=27 because #y=(5^2+2)=27#. We can plug in #x# directly to find the limit because the function is defined and continuous there.
To get an idea of an it intuitively means to find a limit on a graph though, you can look at the graph and decide what is happening at #x#=5. When you move to #x#=5 from the right, what is #y# tending to? Well, to 27. Also, when you move to #x#=5 from the left, what is #y# tending it? 27 again. You can think of limits from the right and left as arrows pointing right and left respectively to the #x# value you're looking for. You're kind of trying to "pin point" what is exactly is happening at your graph at that given #x# point. In this case it's quite simply reading the #y# value off the graph, since the left and right limits tend to the same point and therefore are equal.
So,
#lim_(x->5) (x^2+2)=27#


