Question

How do you solve the right triangle ABC given b=14, c=12.2, C=51 degrees?

Answer 1

There is no declaration as to which of opposite, adjacent or hypotenuse is associated with which number. So you have to use known facts to test for 'truth' of relationship. See below:

Explanation:

To investigate if b is the opposite, adjacent or hypotenuse.
Known: size of hypotenuse > opposite and size of hypotenuse > adjacent
As b>c investigate the potential of b being hypotenuse.

Part (A)
If b is the hypotenuse and c opposite then `b sin(51) = c = 12.2` is true ...( 1 )

test `14 sin(51) = 9.383...` ..... ( 2 )

( 2 ) `!=` (1 ) so false

Part(B)
If b is the hypotenuse and c is the adjacent then `b cos(51)=c=12.2` is true.

test `14 cos(51)= 10.390...` ... ( 3 )
( 3 ) `!=` ( 1 ) so also false

Thus the only thing left is for each of b and c to be one of opposite and adjacent.

If angle C is `45^0` then b = c. This is not the case. As angle `C > 45^0` it must be the case that b being the bigger is the opposite

This means that we have:

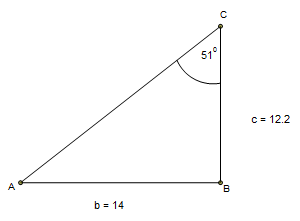
By the way, it is convention for the side opposite the angle to have the same letter as the angle but the angle is upper case and the side lower case!

Now having all the information available it is just a matter of using Pythagoras to solve for the missing length.

I will let you do that using: `(AC)^2 = (AB)^2 + (BC)^2`