Question

The legs of a right triangle are 5cm and 12 cm long. How do you find the lengths, to the nearest tenth, of the segments into which the bisector of the right angle divides the hypotenuse?

Answer 1

Draw a sketch, then use the Law of Sines

Explanation:

Here is a sketch of the problem:

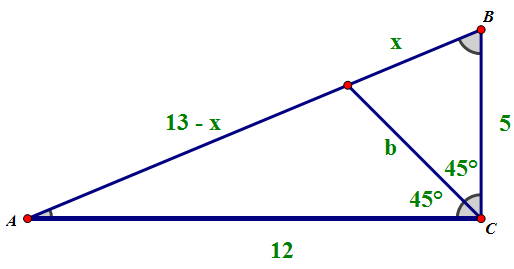
Using Pythagorean theorem, we know the hypotenuse length of `Delta ABC` is 13. So the two segment lengths can be labeled (13-x) and x.

We also know:
`/_A=tan^-1(5/12)`
`/_B=tan^-1(12/5)`

Now use the Law of Sines:

`(sin45)/x=(sin(tan^-1(12/5))/b)`
`(sin45)/(13-x)=(sin(tan^-1(5/12))/b)`

Next, divide the first equation above by the second equation so that both `sin45` and b cancel out.

You will be left with the following identity:

`(13-x)/(x)=(sin(tan^-1(12/5)))/(sin(tan^-1(5/12)))`

Solving for x and (13-x):
`x=3.8`
`13-x=9.2`

Hope that helped