Two corners of a triangle have angles of # ( pi )/ 2 # and # ( pi ) / 6 #. If one side of the triangle has a length of # 4 #, what is the longest possible perimeter of the triangle?

Question

1155 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-09-06T09:43:34

The longest possible perimeter is #18.9282#

As two angles are #pi/2# and #pi/6#, third angle is

#pi-pi/2-pi/6=(6pi)/6-(3pi)/6-pi/6=(2pi)/6=pi/3#

Observe that as the angles are #30^o,60^o,90^o#, the longest side (hypotenuse) is double the smallest side.

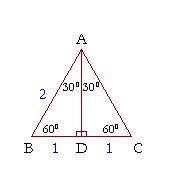
enter image source here

The longest perimeter would be when the side #4# would be opposite smallest angle #pi/6#.

Then longest side of triangle is #2xx4=8# and third side using Pythagoras theorem is

#sqrt(8^2-4^2)=sqrt(64-16)-sqrt48=6.9282#

and longest possible perimeter is #4+8+6.9282=18.9282#