Given the radius of inscribed circle with the angles of the triangle, how do you find the sides given the circle is inscribed the triangle, the radius is 19, sides of triangle was not given only the angles, 17 degrees, 78 degrees, 85 degrees?

Question

4077 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-01-28T08:07:05

The three sides are #150.6#, #147.9# and #44.2#

Let us look at the diagram as follows:
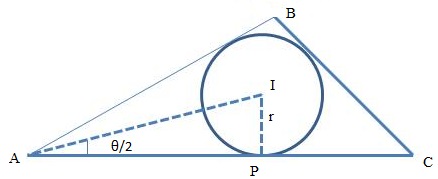
enter image source here

It is apparent that is #m/_A=theta#, #AP=rcot(theta/2)#

and we can similarly calculate #CP=rcot(C/2)#

therefore #AB=AP+CP=r(cot(theta/2)+cot(C/2))#

As the three angles are #17^@#, #78^@# and #85^@# and radius of inscribed circle is #19#,

the three sides are

#19xx(cot8.5^@+cot39^@)=19xx(6.691+1.235)=150.594#

#19xx(cot8.5^@+cot42.5^@)=19xx(6.691+1.091)=147.858#

#19xx(cot39^@+cot42.5^@)=19xx(1.235+1.091)=44.194#

i.e. three sides are #150.6#, #147.9# and #44.2#