How do you write the equation of the parabola in vertex form given vertex at (10, 0) and a directrix x = -2?
2 Answers
Explanation:
As the vertex is
Now parabola is the locus of a point
As distance from directrix is
or
or
graph{(y^2-48x+480)(x+2)((x-10)^2+y^2-0.2)((x-22)^2+y^2-0.2)=0 [-30.33, 49.67, -18.08, 21.92]}
#y^2=48(x-10)#
Explanation:
Look at the diagram -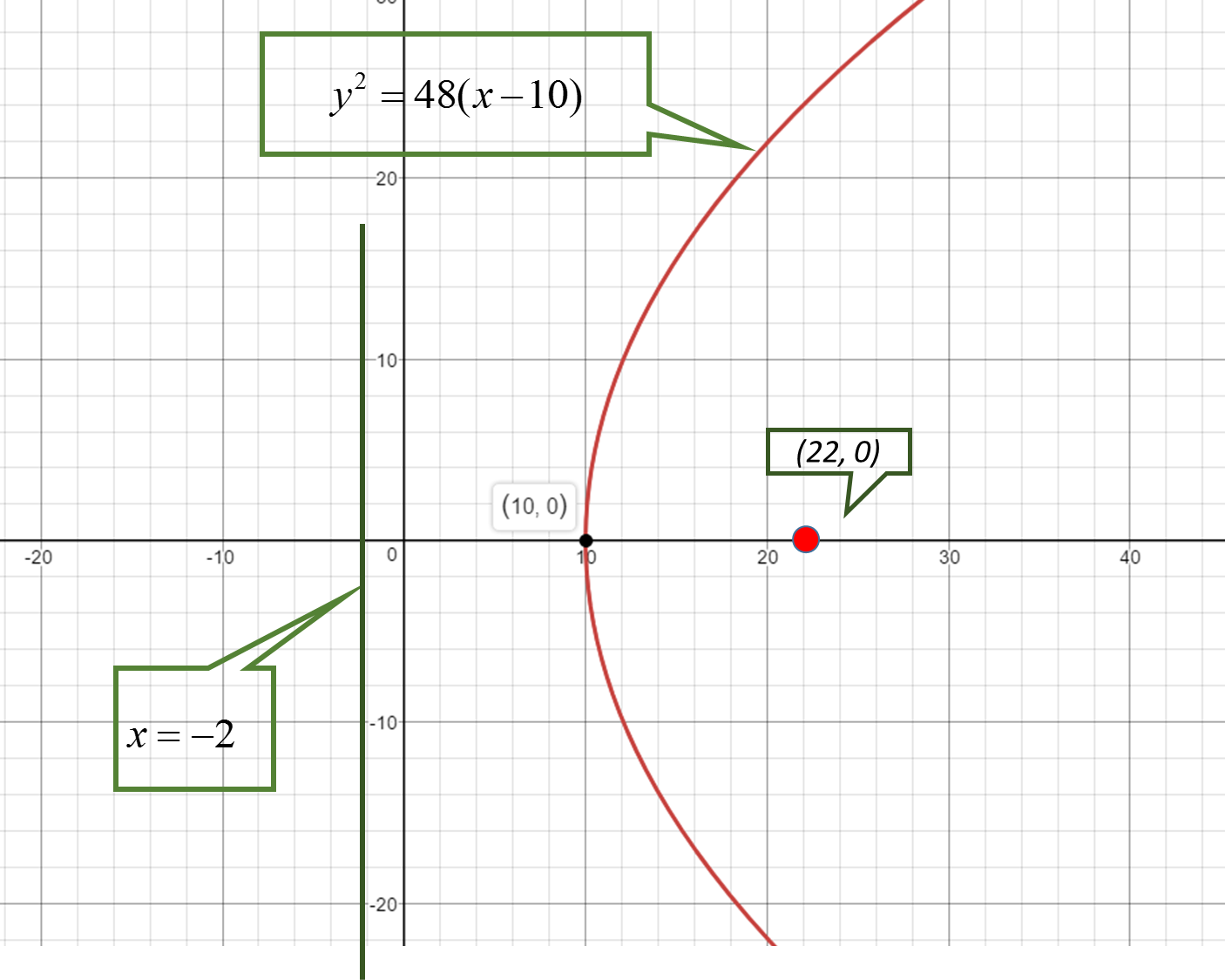
The curve opens to the right, hence its equation is -
#(y-k)^2=4xxaxx(x-h)#
Where-
#h=10# - x-coordinate of the vertex
#k=0# - y-coordinate of the vertex.
#a=12# - distance from the vertex to focus.
#(y-0)^2=4 xx 12xx(x-10)#
#y^2=48(x-10)#

