Question

How do you represent electron orbitals through drawings?

Answer 1

Briefly, drawings reflect the probability density distribution for the wave function outputs for a specific orbital with quantum numbers `n, l, m_l` and `m_s`. They are called contour representations.

Explanation:

Each "drawing" is the probability density of the wave function for each respective sub-shell in a 3d plane.

Firstly, we have four quantum numbers:
`n`: the principal quantum number; defines the size of the orbital
`l`: the angular quantum number; defines the shape of the orbital; each value corresponds to a different shape:

`m_l`: the magnetic quantum number; defines the position in space of the orbital and is derived from a range of `[-l,l]`
`m_s`: the magnetic spin number; defines the rotation of the electron which is a requisite for its magnetic field

For instance, the 2p subshell (`n = 2`, `l = 1`) is larger than a 1s orbital. The p subshell has three orbitals since its angular quantum number `l` is 1, and the magnetic quantum number `m_l` ranges from `[-1, 1]`. Likewise, for the s subshell, its angular quantum number, `l` is 0, which defines its shape, and the magnetic quantum number `m_l` is only 0 which defines its position in space.

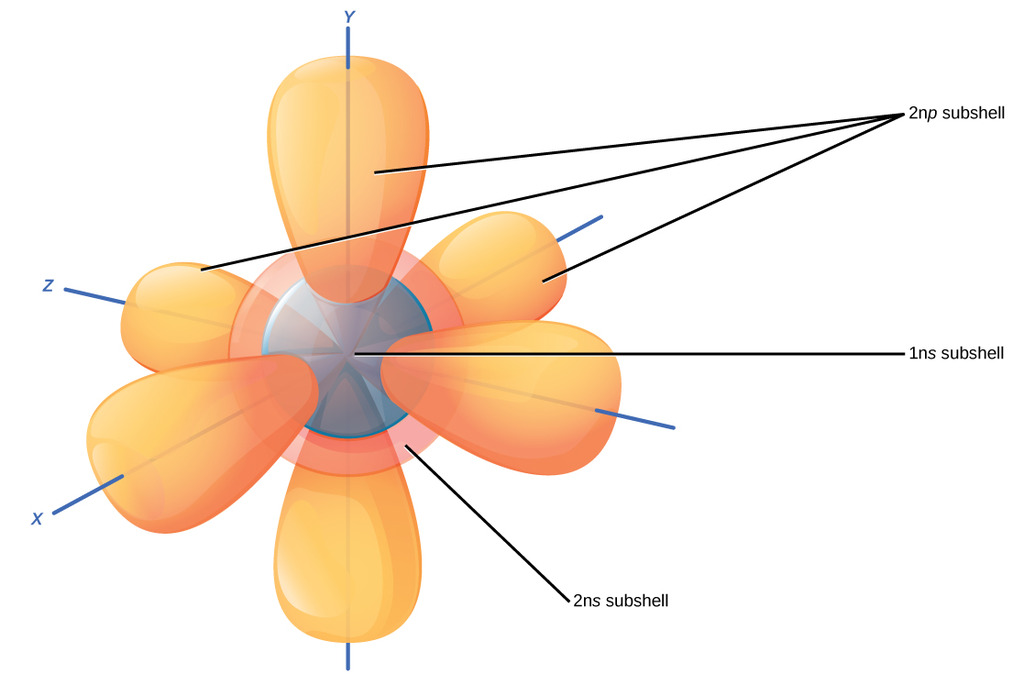
This picture at first seems like a giant, confusing mess. But let's tear it apart. It's merely the 1s, 2s, and 2p subshells for an atom. All of these are where electrons can be located in that atom, for instance this could be `Ne`.

Each of these orbitals in an `Ne` atom contain two electrons. On the first magnitude of distance from the nucleus (at the origin), we have the 1s subshell. Afterwards, we have the 2s subshell, with the 2p subshell containing 3 orbitals since `m_l=-1,0,1`. The probability density (e.g. how likely an electron will be somewhere) increases for p as we're further away from the nucleus, since the electrons in the s subshells will repel the p subshell electrons.

To extrapolate, a 3s subshell would merely be a sphere covering all of these orbitals, with two more electrons, and so on.

Answer 2

I assume you mean in an electron configuration, rather than an actual sketch of the orbitals. They could be represented through horizontal lines, and the electrons through arrows.

The `1s` orbital could be represented as:

`ul(color(white)(uarr darr))`
`1s`

When half-filled, it could be represented as:

`ul(uarr color(white)(darr))`
`1s`

And when filled:

`ul(uarr darr)`
`1s`

Any other orbital can be represented in this way, and the higher up they are (relative to each other), the higher in energy they are.

---

The `uarr` is the spin-up electron (with spin `+1/2`), and the `darr` is the spin-down electron (with spin `-1/2`). By the Pauli Exclusion Principle, no two electron can share the same quantum state.

Being in the same orbital gives two electrons the same principal quantum number `n`, angular momentum quantum number `l`, and magnetic quantum number `m_l` already, but not necessarily `m_s`, their spin.

Therefore, no two electrons can have the same spin in the same orbital.