Question

What is the difference between the graph of `y=2x^2+3x-4` and the graph of `y=2(x-4)^2+3(x-4)-4`?

Answer 1

Let's expand the second function:

`y=2(x^2-8x+16)+3x-12-4`

`= 2x^2-16x+32+3x-16`

`= 2x^2 -13 x + 16`

So, both functions are quadratic polynomials, and then in both cases you have a parabola, and since the `x^2` coefficients are both positive (they are both `2`), they are both `cup`-shaped, instead of `cap`-shaped.

I wouldn't know what to add, they are simply two different parabolas, what did you mean with "what is the difference between" them?

Answer 2

The difference between them is that one is a duplicate of the other but has been shifted to the right by 4

Explanation:

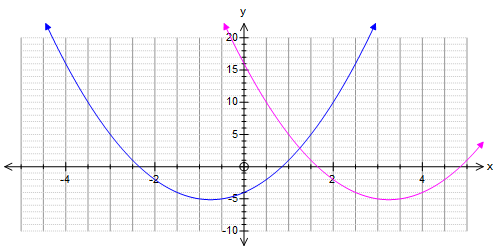
Blue graph is `y=2x^2+3x-4`

It takes the y-value you would have obtained on the blue graph at its `(x-4)` and plotted it at `x`. The consequence of this is as though the whole thing has been 'shunted' to the right on the x-axis by 4