Simple Harmonic Motion - Pendulums
Key Questions
-
The inverse of period equals the frequency:
#f=1/T# 
Michael
·
1
·
Feb 13 2015
-
The frequency is independent of the amplitude.
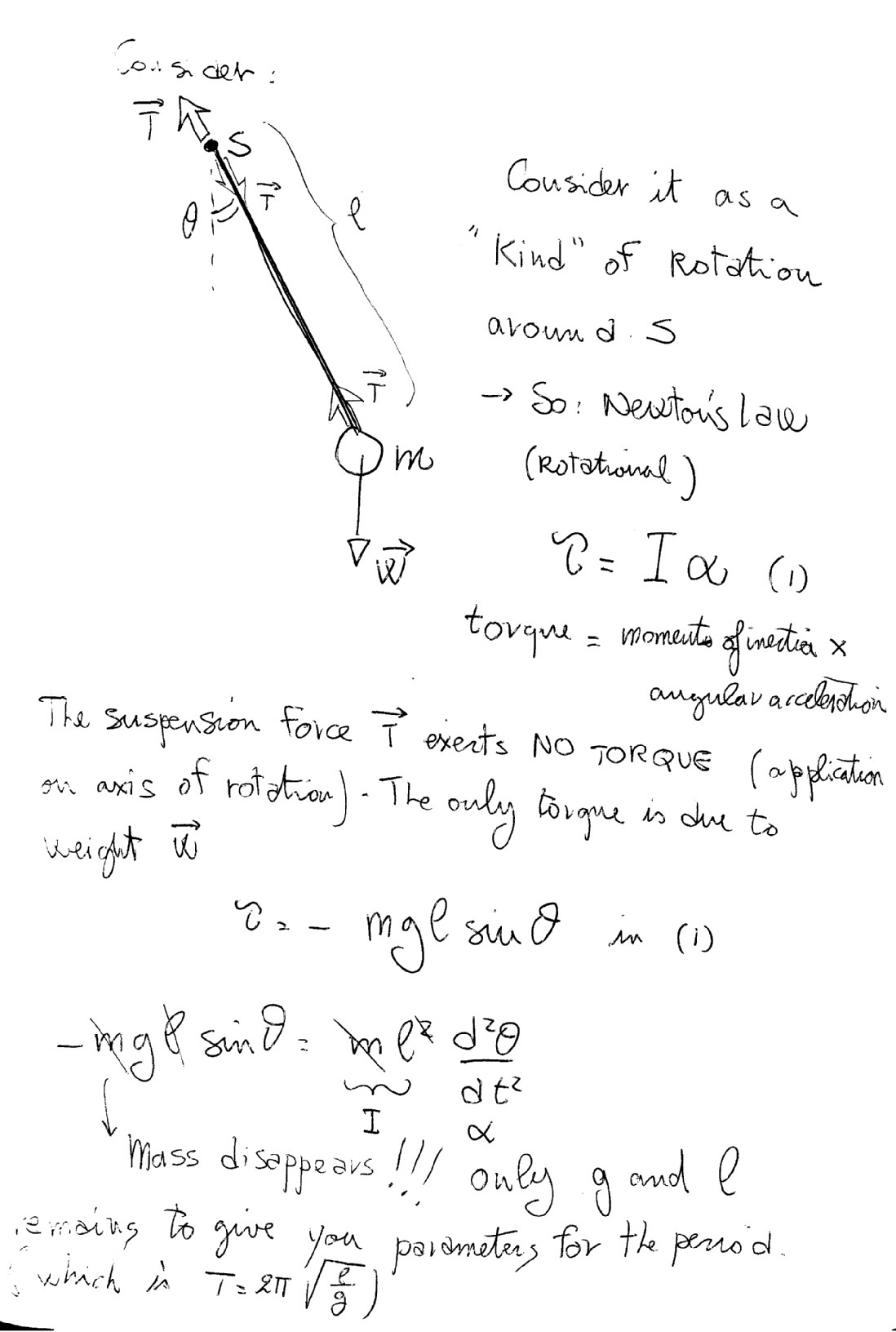
The key equation for SHM is:
#a=–ω^2x#
Where the angular frequency,#ω=2πf#
#a# is the acceleration,
and#x# is the displacement.As you can see the frequency is not related to the amplitude. Actually
#ω# is a constant for this equation. It is the proportionality constant for#aprop-x# . Thus frequency is dependent only on the dimensions of the oscillator (e.g. mass of bob and length of string for pendula or force constant of spring and mass for mass-spring systems). This is the important point here, frequency is independent of amplitude only for a given oscillator. All SHM oscillators do not have the same frequency!The equation,
#a=–ω^2x# , can be proven mathematically but it is quite a long derivation. I will add it below when I have time.In practical situations the presence of friction and drag do cause the frequency to change if amplitude changes, especially at large amplitudes.
Daniel W.
·
·
Jun 12 2014
-
Answer:
They are used for both traditional and modern purposes
Explanation:
Apart from many old-style uses (like clocks or hypnosis, for instance) they are used in many other ways.
Some skyscrapers are built with a huge pendulum inside its upper floors, so that it takes the most of momentum due to the wind. This way, the building structure remains steady.
There are many other purposes which pendulums are used for; a quick search on Google or DuckDuckGo could give a lot of information.
Pendulums' utility is based on conservation of momentum and periodicity of oscillations.P.S. I do not have too much time to answer this question, sorry.
Miguel F.
·
3
·
Sep 25 2015
-
Look, I am not sure this answer is going to satisfy you, but...
This is an "old" derivation (when I was a student....!) so it can be a little bit difficult to digest:
Gió
·
2
·
May 4 2015